next up previous contents index
Next: 定積分の計算(calculation of integrals) Up: 積分法(INTEGRATION) Previous: 無理関数の積分法(integration of irrational functions)   目次   索引

定積分(definite integral)

ここではドイツの数学者 G.F.B. Riemann (1826-1917) によって示されたRiemann積分について学んでいきます．

$ f(x)$ は閉区間 $ [a,b]$ で定義されているとします．この区間 $ [a,b]$ を次のような点 $ x_{i}(i = 1,2,\ldots,n)$ で $ n$ 個の小区間に分割します．

図 3.3: 定積分
\begin{figure}\begin{center} \includegraphics[width=7.1cm]{CALCFIG/Fig3-7-1.eps} \end{center}\vspace{-1cm} \end{figure}

$\displaystyle a= x_{0} < x_{1}<x_{2}<\cdots< x_{i} < \cdots <x_{n} = b $

この分割を $ \Delta$ で表わし， $ \Delta x_{i} = x_{i} - x_{i-1} (i = 1,2,\ldots,n)$ のうちで最も大きい値を $ \vert\Delta\vert$ で表わします．いま，それぞれの小区間 $ [x_{i-1},x_{i}]$ のなかに任意の点 $ \xi_{i}$ をとり，Riemann和(Riemann sum) とよばれる次の和を考えます．

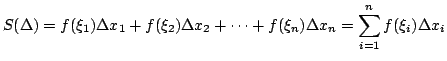
$\displaystyle S(\Delta) = f(\xi_{1})\Delta x_{1} + f(\xi_{2})\Delta x_{2} + \cdots + f(\xi_{n})\Delta x_{n} = \sum_{i=1}^{n}f(\xi_{i})\Delta x_{i} $

このとき，

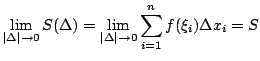
$\displaystyle \lim_{\vert\Delta\vert \rightarrow 0}S(\Delta) = \lim_{\vert\Delta\vert \rightarrow 0}\sum_{i=1}^{n}f(\xi_{i})\Delta x_{i} = S $

となる実数 $ S$ が存在するならば，この $ S$ を $ f(x)$ の 定積分(definite integral) といい， $ f(x)$ は $ [a,b]$ で積分可能(integrable) であるといいます．また，この $ S$ を次のように表わします．

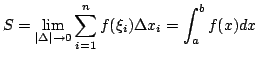
$\displaystyle S = \lim_{\vert\Delta\vert \rightarrow 0}\sum_{i=1}^{n}f(\xi_{i})\Delta x_{i} = \int_{a}^{b}f(x)dx $

つまり関数 $ f(x)$ が $ [a,b]$ で積分可能であるということは，分割の仕方および点 $ \xi_{i}(i = 1,2,\ldots,n)$ のとり方に関係なく一通りに定まるということです．これより，区分求積法とよばれ，次のようにして積分を求める方法があります． $ h = \frac{b-a}{n}$とすると，

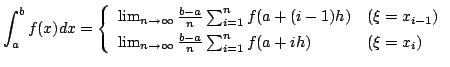
$\displaystyle \int_{a}^{b}f(x) dx = \left\{\begin{array}{ll} \lim_{n \to \infty... ... \infty}\frac{b-a}{n}\sum_{i=1}^{n}f(a + ih) & (\xi = x_{i}) \end{array}\right.$

では，どんな関数が $ [a,b]$ で積分可能になるのでしょうか．次の定理はそんな疑問に答えてくれます．

定理 3..7  

$ f(x)$ が $ [a,b]$ で連続ならば， $ [a,b]$ で積分可能である．


今後，特に断らない限りこの章にでてくる 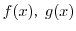$f(x)，g(x)$ などの関数は，考えている区間で連続であるとします．

定積分の定義より，ただちに次の公式が得られます．

定理 3..8  

$ f(x),g(x)$ は区間 $ [a,b]$ で連続であるとすると，

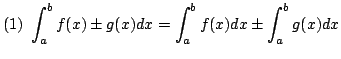
$ \displaystyle{(1) \int_{a}^{b}{f(x) \pm g(x)}dx = \int_{a}^{b}f(x)dx \pm \int_{a}^{b}g(x)dx }$

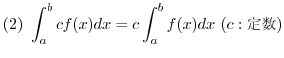
$\displaystyle{(2) \ \int_{a}^{b}cf(x)dx = c\int_{a}^{b}f(x)dx \ (c : \mbox{定数})}$

$ \displaystyle{(3) \int_{a}^{b}f(x) dx = - \int_{b}^{a} f(x) dx }$

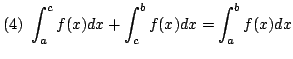
$ \displaystyle{(4) \int_{a}^{c}f(x)dx + \int_{c}^{b}f(x)dx = \int_{a}^{b}f(x)dx} $

$\displaystyle{(5) \ [a,b]\mbox{で} \ f(x) \geq g(x) \mbox{ならば，} \ \int_{a}^{b}f(x)dx \geq \int_{a}^{b}g(x)dx }$


証明 (1) $ a < b$ の場合． $ [a,b]$ の任意の分割を

$\displaystyle a = x_{0} < x_{1} < \cdots < x_{n} = b $

とし， $ \xi_{i}$ を $ [x_{i-1},x_{i}]$ 内の任意の点としてRiemann和を考えると，

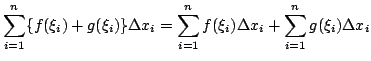
$\displaystyle \sum_{i=1}^{n}\{f(\xi_{i}) + g(\xi_{i})\}\Delta x_{i} = \sum_{i=1}^{n}f(\xi_{i})\Delta x_{i} + \sum_{i=1}^{n}g(\xi_{i})\Delta x_{i} $

ここで， $ \vert\Delta\vert \rightarrow 0$ とすると，定理3.7より，

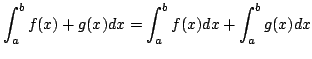
$\displaystyle \int_{a}^{b}{f(x) + g(x)}dx = \int_{a}^{b}f(x)dx + \int_{a}^{b}g(x)dx $

(3)
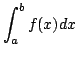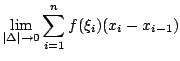$\displaystyle \int_{a}^{b} f(x) dx$ $\displaystyle =$ $\displaystyle \lim_{\vert\Delta\vert \rightarrow 0}\sum_{i=1}^{n} f(\xi_{i})(x_{i} - x_{i-1})$  
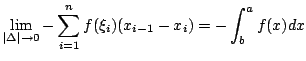  $\displaystyle =$ $\displaystyle \lim_{\vert\Delta\vert \rightarrow 0} - \sum_{i=1}^{n} f(\xi_{i})(x_{i-1} - x_{i}) = - \int_{b}^{a}f(x) dx$  

(2)，(4)，(5)の証明は各自に任せます． $ \blacksquare$

微分積分学の基本定理

関数 $ f(x)$ が $ [a,b]$ で連続であるとき， $ [a,b]$ 内の任意の点 $ x$ に対して定積分 $ \int_{a}^{x}f(t)dt$ を考えると，これは $ [a,b]$ を定義域にもつ関数になります．この関数について，次の定理が成り立ちます．

定理 3..9  

$ f(x)$ が $ [a,b]$ で連続であれば， $ \int_{a}^{x}f(t)dt$ は $ x$ について微分可能であって，

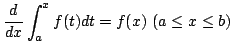
$\displaystyle \frac{d}{dx}\int_{a}^{x}f(t)dt = f(x) (a \leq x \leq b) $

となる．


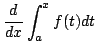証明 $ \displaystyle{F(x) = \int_{a}^{x}f(t)dt}$ とおくと， $ \displaystyle{\frac{d}{dx}\int_{a}^{x}f(t)dt}$ は $ F(x)$ の導関数を求めることになるので， $ \displaystyle{\frac{F(x+h) -F(x)}{h}}$ を考えます．まず， $ h > 0$ のとき，

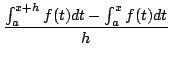$\displaystyle \frac{F(x+h) -F(x)}{h}$ $\displaystyle =$ $\displaystyle \frac{\int_{a}^{x+h}f(t)dt - \int_{a}^{x}f(t)dt}{h}$  
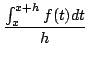  $\displaystyle =$ $\displaystyle \frac{\int_{x}^{x+h}f(t)dt}{h}$  

ここで $ M_{h}$ を $ [x,x+h]$ における $ f(x)$ の最大値， $ m_{h}$ を $ [x,x+h]$ における $ f(x)$ の最小値とすると(なぜ最大値，最小値が存在するとわかる?)， $ [x,x+h]$ で

$\displaystyle m_{h} \leq f(x) \leq M_{h} $

したがって，定理3.7より

$\displaystyle m_{h}[(x+h)-x] \leq \int_{x}^{x+h}f(t)dt \leq M_{h}[(x+h) - x] $

となる．そこで，両辺を $ h$ でわると， 仮定より， $ f(x)$ は $ [x,x+h]$ で連続なので，

$\displaystyle \lim_{h \rightarrow 0+}m_{h} = f(x) = \lim_{h \rightarrow 0+}M_{h} $

よって，はさみうちの定理より

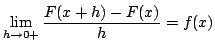
$\displaystyle \lim_{h \rightarrow 0+}\frac{F(x+h) -F(x)}{h} = f(x) $

同様に $ h < 0$ のとき

$\displaystyle \lim_{h \rightarrow 0-}\frac{F(x+h) -F(x)}{h} = f(x) $

となり， $ F(x)$ は微分可能であり， $ F^{\prime}(x) = f(x)$ となる． $ \blacksquare$

この定理より， $ F(x) = \int_{a}^{x}f(t)dt$ は $ f(x)$ の原始関数となります．よって，連続な関数は必ず原始関数をもっていることがわかり，その原始関数は定積分で与えられます．

例題 3..21  

$ f(t)$ が連続のとき

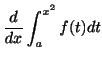
$\displaystyle \frac{d}{dx}\int_{a}^{x^{2}}f(t)dt $

を求めてみましょう．

解 まず， $ u = x^2$ とおくと $ du = 2x dx$．よって

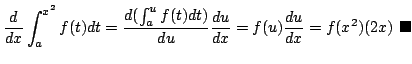
$\displaystyle \frac{d}{dx}\int_{a}^{x^{2}}f(t)dt = \frac{d (\int_{a}^{u} f(t) dt)}{du}\frac{du}{dx} = f(u)\frac{du}{dx} = f(x^2)(2x) \ensuremath{ \blacksquare}$

定理 3..10  

[微分積分学の基本定理] 閉区間 $ [a,b]$ で連続な関数 $ f(x)$ の $ 1$つの原始関数を $ G(x)$ とすると

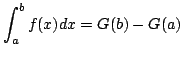
$\displaystyle \int_{a}^{b}f(x)dx = G(b) - G(a) $

である．


証明 定理3.7より， $ F(x) = \int_{a}^{x}f(t)dt$ も $ f(x)$ の原始関数です．よって，定理3.1より $ F(x) = G(x) + c (c :$   定数$ )$. ここで $ F(a) = 0$ だから， $ F(a) = G(a) + c = 0$．よって， $ c = -G(a)$. このとき $ F(x) = G(x) - G(a)$ となるから， $ x = b$ とすると，

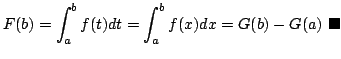
$\displaystyle F(b) = \int_{a}^{b}f(t)dt = \int_{a}^{b}f(x)dx = G(b) - G(a) \ensuremath{ \blacksquare}$

ここで， $ G(b) - G(a)$ は $ G(x)\vert _{a}^{b}$ または $ [G(x)]_{a}^{b}$ と略記されます．

この定理より，定積分の計算は被積分関数の不定積分を求め，積分範囲の端点を代入しその差を求めればよいことがわかります．

例題 3..22  

次の定積分の値を求めてみましょう．

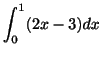
$\displaystyle \int_{0}^{1}(2x - 3)dx$

解

$\displaystyle \int_{0}^{1}(2x -3)dx = \left[x^2 - 3x \right]_{0}^{1} = 1 - 3 = -2 \ensuremath{ \blacksquare}$

確認問題


1.
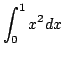区分求積法を用いて， $ \displaystyle{\int_{0}^{1}x^{2}dx}$を求めよう．
2.
次の定積分を計算しよう．

(a) $ \displaystyle{\int_{0}^{1}(x^{2} + 3) dx}$ (b) $ \displaystyle{\int_{1}^{2}\frac{x^{2} - 1}{x} dx}$ (c) $ \displaystyle{\int_{0}^{1}\sqrt{x^{3}} dx}$ (d) $ \displaystyle{\int_{0}^{\pi}\cos{x} dx}$ (e) $ \displaystyle{\int_{0}^{\frac{\pi}{2}} \sin{x} dx}$

3.
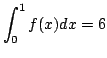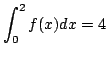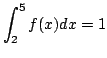$ \displaystyle{\int_{0}^{1}f(x)dx = 6}$, $ \displaystyle{\int_{0}^{2}f(x)dx = 4}$, $ \displaystyle{\int_{2}^{5}f(x)dx = 1}$のとき，次の問いに答えよう．

(a) $ \displaystyle{\int_{0}^{5}f(x) dx}$ (b) $ \displaystyle{\int_{1}^{2}f(x) dx}$ (c) $ \displaystyle{\int_{1}^{5}f(x) dx}$ (d) $ \displaystyle{\int_{0}^{0}f(x) dx}$

(e) $ \displaystyle{\int_{2}^{0}f(x) dx}$

4.
関数 $ f(t)$ が連続であるとき， $ g(x)$ を求めよう．

(a) $ \displaystyle{g(x) = \frac{d}{dx}\int_{1}^{x}\sin{t}dt}$ (b) $ \displaystyle{g(x) = \frac{d}{dx}\int_{x}^{1}\cos{t}dt}$ (c) $ \displaystyle{g(x) = \frac{d}{dx}\int_{0}^{2x}\sqrt{\sin{t}}dt}$

5.
次の極限値を求めよう．

(a) $ \displaystyle{\lim_{n \rightarrow \infty} \frac{1}{n}\left(\frac{1}{n} + \frac{2}{n} + \cdots + \frac{n}{n} \right)}$ (b) $ \displaystyle{\lim_{n \rightarrow \infty} \frac{1}{n}\left(\frac{1}{2 + \frac{1}{n}} + \frac{1}{2 + \frac{2}{n}} + \cdots + \frac{1}{3} \right)}$

(c) $ \displaystyle{\lim_{n \rightarrow \infty} \frac{1}{n}\sum_{i=1}^{n}\sqrt{\frac{i}{n}}}$

演習問題


1.
関数 $ f(t)$ が連続であるとき， $ g(x)$ を求めよう．

(a) $ \displaystyle{g(x) = \frac{d}{dx}\int_{x}^{b}f(t)dt}$ (b) $ \displaystyle{g(x) = \frac{d}{dx}\int_{x}^{x+1}f(t)dt}$

(c) $ \displaystyle{g(x) = \frac{d}{dx}\int_{0}^{2x}x^{2}f(t)dt}$

2.
次の定積分を計算しよう．

(a) $ \displaystyle{\int_{1}^{5}2\sqrt{x-1}dx}$ (b) $ \displaystyle{\int_{1}^{2}\frac{2-t}{t^{3}}dt}$ (c) $ \displaystyle{\int_{0}^{\frac{\pi}{2}}\cos{x}dx}$ (d) $ \displaystyle{\int_{0}^{1}xe^{-x^{2}}dx}$

(e) $ \displaystyle{\int_{0}^{\log{2}} \frac{e^{x}}{e^{x} + 1}dx}$

3.
定理3.7(2)，(3)，(4)，(5)を証明しよう．
4.
次の不等式を証明しよう．

(a) $ \displaystyle{\frac{\pi}{4} < \int_{0}^{1}\frac{1}{1 + x^n}dx < 1 (n > 2)}$ (b) $ \displaystyle{\frac{1}{2n+2} \leq \int_{0}^{1} \frac{x^n}{1 + x}dx \leq \frac{1}{n} (n \geq 1)}$

5.
次の極限値を求めよう．

(a) $ \displaystyle{\lim_{n \rightarrow \infty} \left(\frac{1}{n+1} + \frac{1}{n + 2} + \cdots + \frac{1}{2n} \right)}$ (b) $ \displaystyle{\lim_{n \rightarrow \infty} \sum_{i = 1}^{n} \sqrt{\frac{1}{n^2 + i^2}}}$

(c) $ \displaystyle{\lim_{x \rightarrow 0} \int_{0}^{x} \tan{(t^2)}dt}$

next up previous contents index
Next: 定積分の計算(calculation of integrals) Up: 積分法(INTEGRATION) Previous: 無理関数の積分法(integration of irrational functions)   目次   索引