Next: 積分法(INTEGRATION) Up: 微分法(DIFFERENTIATION) Previous: 不定形の極限値(limit of indeterminate forms) 目次 索引
超越関数 ![]() を多項式を用いて表わすことができないでしょうか.もしそうなれば ,整関数さえ知っていれば他の関数のことを知らなくてすむのです.そんな疑問に イギリスの数学者 Brook Taylor (1685-1731) は1712年に答えてくれました.
を多項式を用いて表わすことができないでしょうか.もしそうなれば ,整関数さえ知っていれば他の関数のことを知らなくてすむのです.そんな疑問に イギリスの数学者 Brook Taylor (1685-1731) は1712年に答えてくれました.

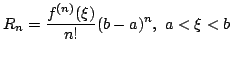
証明
定数 ![]() を
を
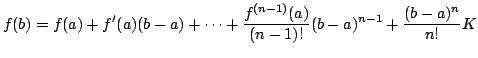
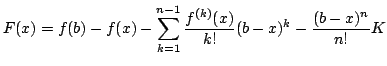
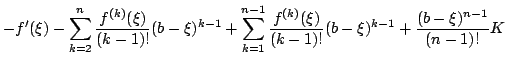 |
|||
 |
ここで用いられた ![]() を Lagrangeの剰余数(Lagrange's remainder) といい,
を Lagrangeの剰余数(Lagrange's remainder) といい,

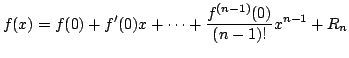
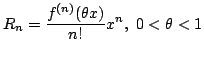
ここで誤差の評価は
![$\displaystyle \vert R_{n}\vert = \vert\frac{f^{(n)}(\theta x)}{n!}x^{n}\vert \l...
...max_{\theta \in [0,1]}\vert f^{(n)}(\theta x)\vert)\frac{\vert x\vert^{n}}{n!} $](img1768.gif)
解
![]() より
より
![]() よって
よって

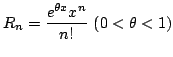 が成り立ちます.ところが,
が成り立ちます.ところが,
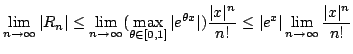
![]() は
は ![]() を含む区間
を含む区間 ![]() で
で
![]() 級関数とすると,Maclaurinの定理より任意の自然数
級関数とすると,Maclaurinの定理より任意の自然数 ![]() に対して,
に対して,

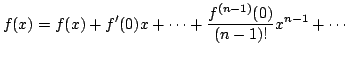
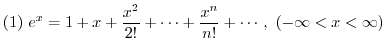
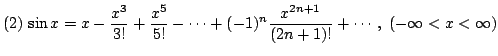
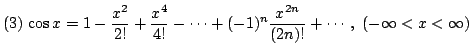
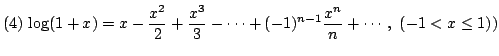

ただし,
![]()
証明 (1)はすでに例題で行いました.
(2)
![]() とすると
とすると
![]() より
より
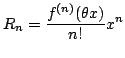

ここでE.G.H.Landau(1877-1938)によって用いられたちょっと便利な記号を紹介します.例えば関数 ![]() の間に
の間に
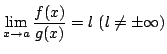
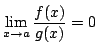
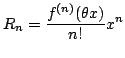
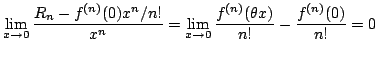

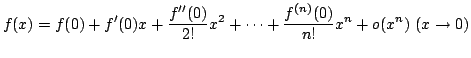
解
![]() のMacLaurin展開より
のMacLaurin展開より
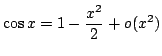 と表わせます.
よって
と表わせます.
よって
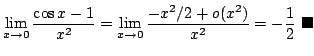
(c)
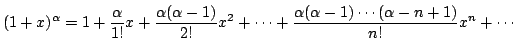
ただし,
![]()
(d)
 ,
,
![]()
(a) 演習問題1(d)より,
![]() を得ることができる.これを用いて,プログラムを組み
を得ることができる.これを用いて,プログラムを組み![]() を小数点以下2桁まで求めてみよう.
を小数点以下2桁まで求めてみよう.
(b)
![]() と表した式をMachinの公式という.この公式を用いて
と表した式をMachinの公式という.この公式を用いて![]() を小数点以下100桁まで求めてみよう.
を小数点以下100桁まで求めてみよう.