Next: Integral formula Up: Scalar field, vector field Previous: Divergence Contents Index
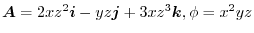 .Find the followings..
.Find the followings..
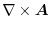
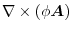
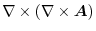
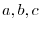 so that
so that
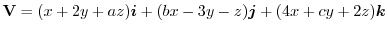 satisfies
satisfies
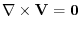 .
.
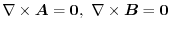 ,then prove that
,then prove that
 .
.
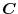 be a constant vector.Then prove the following equation for arbitray vecot field
be a constant vector.Then prove the following equation for arbitray vecot field
 .
.
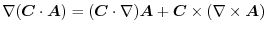
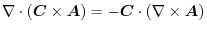
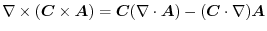
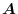 , prove the following.
, prove the following.
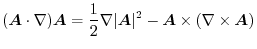
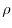 and
and 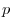 be scalar fields.If
be scalar fields.If
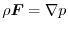 ,then prove that
,then prove that
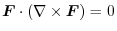 .
.
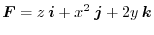
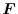 is conservative, show that
is conservative, show that
 .
.
9. Let
 and
and
 be a constant vector, prove the followings..
be a constant vector, prove the followings..
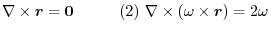

10. Find
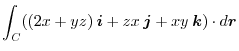 . for
. for
 is a close curve
is a close curve
 is a curve connecting from the point
is a curve connecting from the point
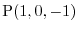 to the point
to the point
 .
.