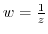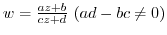Linear function

Let $a,b,c,d$ be complex constants. The function of the form

$$w = \frac{az + b}{cz + d} (ad - bc \neq 0)$$

is called fractional transformation.

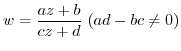
When this function is decomposed, it can be seen that it is a composition of the following three functions.

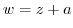• $w = z + a$ Translation on the complex plane
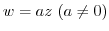• $w = az (a \neq 0)$ rotation and expansion on the complex plane
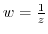• $w = \frac{1}{z}$ Straight lines that pass through the origin are converted into straight lines that pass through the origin, other straight lines are converted into circles that pass through the origin, circles that pass through the origin are converted into straight lines, and other circles are converted into circles.

Exercise2.2

1. What straight line or circle is the image of the next straight line or circle mapped by $w = \frac{1}{z}$.

(a)

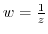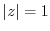A straight line that intersects the unit circle $\vert z\vert = 1$ at two points P and Q

(b)

A straight line tangent to the unit circle at one point P

(c)

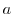A circle passing through 3 points $a$ (real number), $i$, $-i$

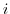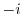
2. Find the invariant point by the following linear transformation on the complex plane.

(a)

$w = \frac{1}{z}$

(b)

$w = \frac{az + b}{cz + d} (ad -bc \neq 0)$