Next: Cauchy's integral theorem Up: Complex integration Previous: Line integral and Green's Index
 be the region containing the curve
be the region containing the curve  on the
on the  plane, and let
plane, and let  be a continuous function defined on
be a continuous function defined on  so that
so that
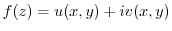
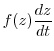 |
 |
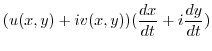 |
|
 |
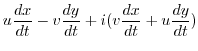 |
 , the integral
, the integral

 and it is a line integral of
and it is a line integral of 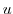 along the curve
along the curve  by
by 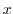 . Thinking about the remaining terms in the same way, the next integral can be considered.
. Thinking about the remaining terms in the same way, the next integral can be considered.
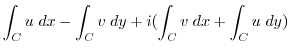
 of the function
of the function  and expressed by
and expressed by

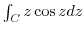 along the curve
along the curve  that goes around the unit circle.
that goes around the unit circle.
2 Integrate the function  for along the sides and diagonals of a square with vertices at point
for along the sides and diagonals of a square with vertices at point
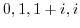 from 0 to
from 0 to 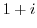 .
.