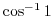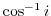Next: Holomorphic function Up: Complex functions Previous: Exponential function Index
 and
and 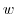 , there is a relationship so that
, there is a relationship so that

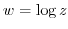
 ,
,
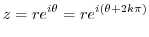
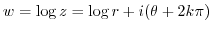
 corresponds to an infinite number of different values 窶鞠or one complex number
corresponds to an infinite number of different values 窶鞠or one complex number  . That is, it is an infinite multivalued function. The principal value or principal brunch of
. That is, it is an infinite multivalued function. The principal value or principal brunch of  is given by
is given by
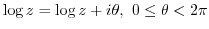
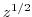 has two branches.
has two branches.
2. Find all of the following values.
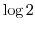
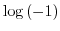

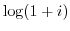
3. Express the following value in the form of 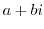 .
.
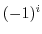
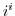
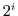

4. Prove the following formulas.

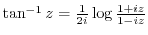
(5) Find the following values.