Next: Taylor's Theorem Up: Differentiation Previous: Mean Value Theorem Contents Index
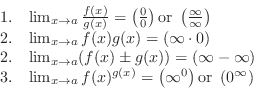
 and
and 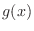 be continuous on
be continuous on ![$[a,b]$](img1050.png) adn differentiable on
adn differentiable on 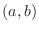 . If
. If
 and
and
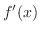 and
and
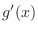 never takes 0 simultaneously, then there exists at leat one number
never takes 0 simultaneously, then there exists at leat one number
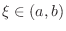 such that
such that

This is wrong Apply the Mean Value Theorem to  and and 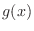 . Then . Then
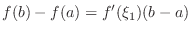
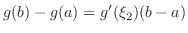
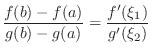
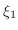 and and 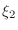 are equal. are equal.
|
| Check |
|---|
 . Then . Then
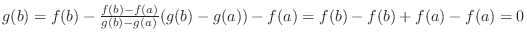 . .
|
We consider a function which satisfies the conditions of Rolle's Theorem. Let
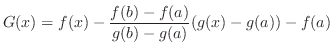
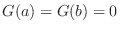 and
and 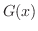 satisfies the conditions of Rolle's Theorem. Thus there exists at least one number
satisfies the conditions of Rolle's Theorem. Thus there exists at least one number
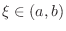 such that
such that
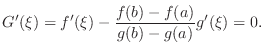
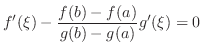
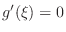 , then
, then
 and this violates the assumption. Therefore,
and this violates the assumption. Therefore,
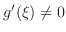 and
and
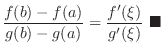
L'Hospital's Theorem
 and
and  be continuous on
be continuous on ![$[a,b]$](img1050.png) and differentiable on
and differentiable on 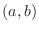 . If
. If
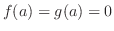 and
and
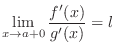 exists, then
exists, then
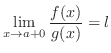 .
.
L'Hospital's Theorem 1. First make sure that limit is in indeterminate form of either
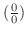 or
or
 .
.
2. Differentiate the numerator and denominator separetely.
3. After differentiation, Simplify the expression.
4. If it is indeterminate form again, repeat 2.3.
Proof
Let  be such that
be such that 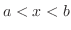 and consider
and consider
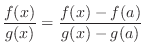
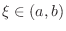 such that
such that
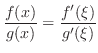
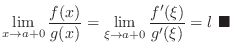
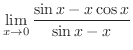
SOLUTION
This is indeterminate form of
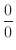 . Then differentiate the numerator and denominator separately, we have
. Then differentiate the numerator and denominator separately, we have
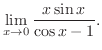
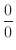 . So, differentiate the numerator and denominator separately, we have
. So, differentiate the numerator and denominator separately, we have

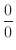 . So, differentiate the numerator and denominator separately, we have
. So, differentiate the numerator and denominator separately, we have

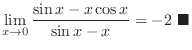
| Check |
|---|
 . .
|
To find the limit by L'Hospital's Theorem, we usually write in the following way.
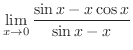 |
 |
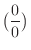 |
|
 |
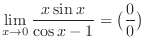 |
||
 |
 |
| Symbol |
|---|
In this paper, when we apply L'Hospital's rule, we use the following symbol
 . .
|
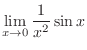
Other than
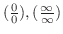 Note that L'Hospital's theorem only can apply
Note that L'Hospital's theorem only can apply
 . Other indeterminate form appears, you must change into
. Other indeterminate form appears, you must change into
 .
.
1.
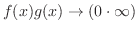


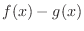 |
|||
 |
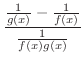 |
||
 |
 |
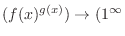 or
or
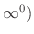
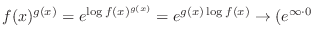 or
or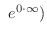
Note that this is indeterminate form of
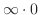 . Then we replace
. Then we replace
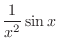 by
by
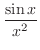 . Then it is indeterminat form of
. Then it is indeterminat form of
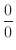 . Thus by L'Hospital's Theorem, we have
. Thus by L'Hospital's Theorem, we have
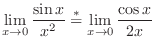
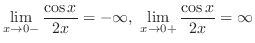 . Thus no limit exists
. Thus no limit exists

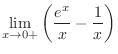
SOLUTION
This is indeterminate form of
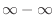 . Then replace
. Then replace
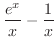 by
by
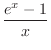 . Then it is indeterminate form of
. Then it is indeterminate form of
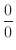 . Thus
. Thus
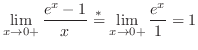
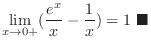
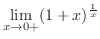
SOLUTION
This is indeterminate form of
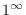 . So we rewrite
. So we rewrite
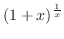 into
into
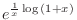 . Then
. Then
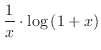 is indeterminate form of
is indeterminate form of
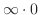 . Thus replace
. Thus replace
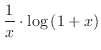 by
by
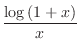 . Then in the form of
. Then in the form of
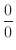 . Thus
. Thus
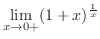 |
 |
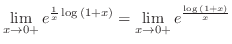 |
|
 |
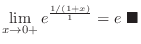 |
|
Exercise A
|
|
Exercise B
|