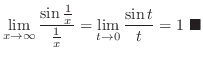Next: One sided limit Up: Functions Previous: Limit of Sequence Contents Index
Intuitive approach to limit
As  approaches
approaches 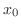 ,
,  approaches
approaches 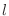 , Then we say
, Then we say 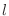 is the limit of
is the limit of  as
as  approaches
approaches 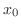 and denote
and denote
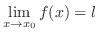
Expression of limit
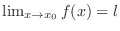 or
or
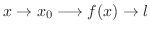 .
.
NOTE  approaches
approaches 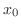 is the same as
is the same as
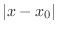 approaches 0. Similarly,
approaches 0. Similarly,  approaches
approaches  is the same as
is the same as
 approaches 0.
approaches 0.
Understanding of limit The concept of infinitesimal can be explained by saying that every small number you choose, we can choose smaller number.
Limit properties
NOTE Limit of functions obey the four rules of arithmetic provided the denominator is not 0.
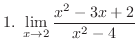
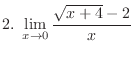
Note that
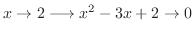 and
and
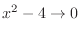 . In other words, both the denominator and the numerator have the common factor
. In other words, both the denominator and the numerator have the common factor 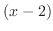 .
.
SOLUTION1.
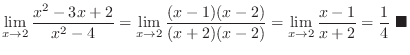 |
As  , we have
, we have
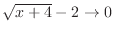 and
and  . Thus we can factorby
. Thus we can factorby  .
.
2.
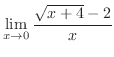 |
 |
 |
|
 |
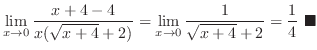 |
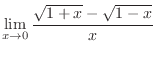
The function
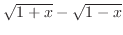 can be rationalize by multiplying
can be rationalize by multiplying
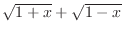 to the both numerator and denominator.
to the both numerator and denominator.
SOLUTION
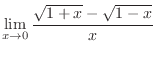 |
|||
 |
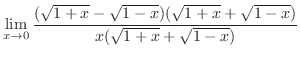 |
||
 |
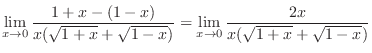 |
||
 |
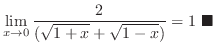 |
Diverges to infinity
We write
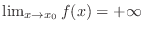 when
when  gets larger without bound as
gets larger without bound as  approaches
approaches 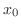 .
We write
.
We write
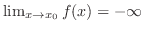 when the value of
when the value of  is negative and the absolute value gets larger without bound as
is negative and the absolute value gets larger without bound as  approaches
approaches 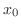 .
.
Difference of two squares
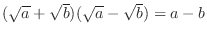 .
.
NOTE  gets larger without bound means that given any large number
gets larger without bound means that given any large number 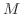 , there exists number
, there exists number 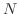 such that
such that 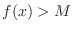 as
as  gets larger than
gets larger than 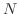 .
We write
.
We write
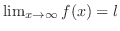 when
when  approaches
approaches 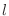 as
as  gets larger without bound.
gets larger without bound.
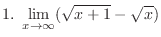
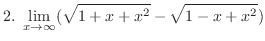
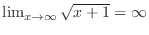 ,
,
 . Thus it is indeterminate form of
. Thus it is indeterminate form of
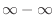 .
.
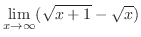 |
 |
 |
|
 |
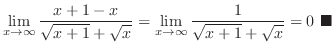 |
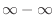 The indeterminate form of
The indeterminate form of
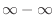 has to be rewrite in the indeterminate form of
has to be rewrite in the indeterminate form of
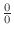 or
or
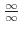 .
.
2.
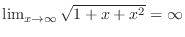 ,
,
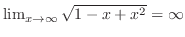 . Thus it is indeterminate form of
. Thus it is indeterminate form of
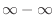 . Now rationalize the fraction
. Now rationalize the fraction
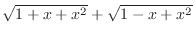 .
.
 |
|||
 |
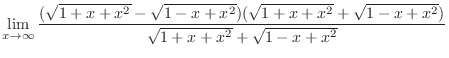 |
||
 |
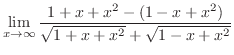 |
||
 |
 |
||
 |
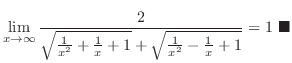 |
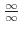 |
|---|
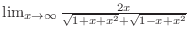 is in the indeterminate form of is in the indeterminate form of
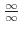 . Then factor the fraction by dividing the largest power of . Then factor the fraction by dividing the largest power of  . .
|

Handling
 If
If
 , then we write
, then we write 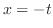 and solve the question.
and solve the question.
SOLUTION Put 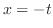 . Then
. Then
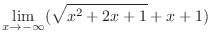 |
 |
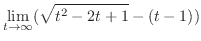 |
|
 |
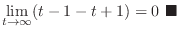 |
Exercise1.22
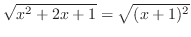 . Note that
. Note that 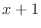 is negative. Thus
is negative. Thus
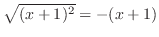 .
.
To find the limit of function, the above theorem is not enogh. For example
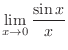 can not be found..
can not be found..
Squeezing theorem
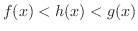 is satisfied for the
is satisfied for the 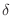 neighborhood
neighborhood
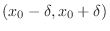 of
of 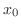 and
and
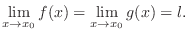
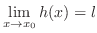 .
.
NOTE
Since
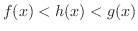 ,
,
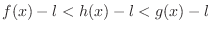 . Note that
. Note that
 and
and
 can be made as small as possible. Thus we can make
can be made as small as possible. Thus we can make
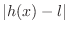 as small as possible .
as small as possible .
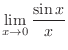 .
.
SOLUTION
Take points
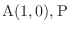 on the unit circle. Now find the intersection of the extended line OP and the line perpendicular to the line OA. We name the intersection B. Also, start from P, find the intersection of the line perpendicular to OA, we name this C. Now we compare the size of the triangles. Then
on the unit circle. Now find the intersection of the extended line OP and the line perpendicular to the line OA. We name the intersection B. Also, start from P, find the intersection of the line perpendicular to OA, we name this C. Now we compare the size of the triangles. Then
 .
Now for
.
Now for
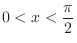 , we have
, we have
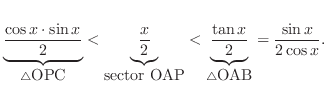
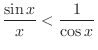 , and the second inequality
, and the second inequality
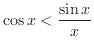 .
Thus
.
Thus
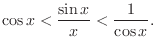
 .
Now we have
.
Now we have
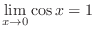 and
and
 . Therefore,
. Therefore,
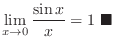
Area of sector We compare the area of sector with the radius 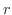 and the angle
and the angle 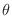 with the area of circle with the radius
with the area of circle with the radius 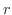 . Then the arc length of the sector is
. Then the arc length of the sector is 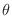 . Thus the area of sector is
. Thus the area of sector is
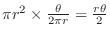 .
.
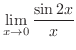 .
. 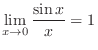 imples that for
imples that for  small,
small, 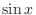 and
and  is about the same.
is about the same.
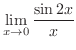 |
 |
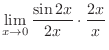 |
|
 |
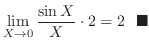 |
Set 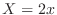 . Then
. Then
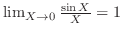 .
.
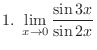
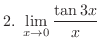
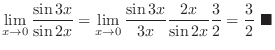
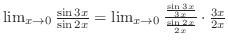 .
.
2.
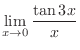 |
 |
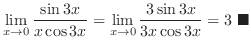 |
Express 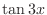 using
using 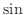 and
and  . Thne
. Thne
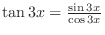
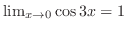 .
.
 .
. 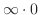 If you have the indeterminate form
If you have the indeterminate form
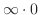 , then express the one of the expression by fraction. Then we can transform into
, then express the one of the expression by fraction. Then we can transform into
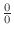 or
or
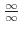 .
SOLUTION As
.
SOLUTION As
 ,
,
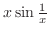 has the indeterminate form
has the indeterminate form
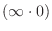 . Then we make this into the indeterminate form of
. Then we make this into the indeterminate form of
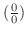 .
.
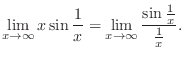
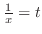 . Then as
. Then as
 , we have
, we have 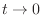 . Thus,
. Thus,