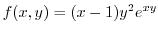Next: 6.5 Answer Up: PARTIAL DIFFERENTIATION Previous: 6.4 Answer 索引
|
Exercise
|
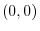 in the direction of
in the direction of
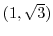 .
.
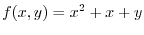
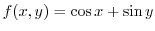
 in the direction of
in the direction of
 .
.
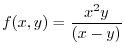
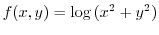
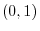 in the direction of
in the direction of 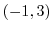 .Find the directional unit vector so that the directional derivatives becomes the maximum.
.Find the directional unit vector so that the directional derivatives becomes the maximum.