Next: 部分積分法(integration by parts) Up: 積分法(INTEGRATION) Previous: 不定積分(indefinite integrals) 目次 索引
不定積分
![]() を求めるときに,被積分関数
を求めるときに,被積分関数 ![]() の原始関数が四則の演算により積分公式(1)から(12)の形に直せないことがよくあります.そんなとき,
の原始関数が四則の演算により積分公式(1)から(12)の形に直せないことがよくあります.そんなとき, ![]() の
の ![]() をある
をある ![]() の関数
の関数 ![]() に置き換えることにより,
に置き換えることにより, ![]() を
を
![]() という,公式(1)から(12)を用いることにより積分できる形に変形することを 置換積分法(integration by substitution) といいます.
という,公式(1)から(12)を用いることにより積分できる形に変形することを 置換積分法(integration by substitution) といいます.
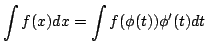
証明
![]() とすると,
とすると,
![]() である.そこで
である.そこで
![]() とおき,
とおき,
![]() を考えると,
を考えると,
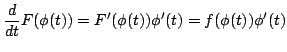
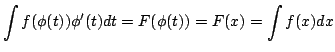
この定理により,
![]() を求めるのに,
を求めるのに,
![]() とその微分
とその微分
![]() とをもとの式に形式的に代入し,
とをもとの式に形式的に代入し,
![]() としてこれを計算すればよいことがわかります.
としてこれを計算すればよいことがわかります.
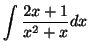 を求めてみましょう.
を求めてみましょう.
解
このままの形では,公式(1)から(12)のどれも使えないことがわかります.そこで
![]() とおきます.すると
とおきます.すると
![]() となるので,これを用いて元の不定積分を書き直すと
となるので,これを用いて元の不定積分を書き直すと
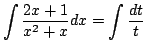
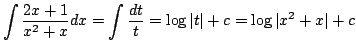
この例題に用いたテクニックを一般化すると次の公式を得ます.
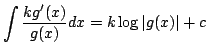
この公式を用いると
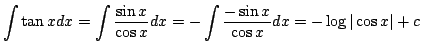
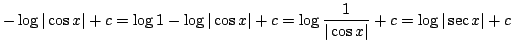
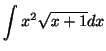 を求めてみましょう.
を求めてみましょう.
解
![]() とおくと,
とおくと, ![]() .また
.また ![]() より,
より,
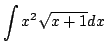 |
 |
||
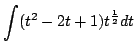 |
|||
 |
|||
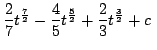 |
|||
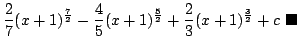 |
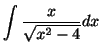 を求めてみましょう.
を求めてみましょう.
解
![]() とおくと,
とおくと,
![]() となるので,
となるので,
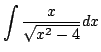 |
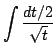 |
||
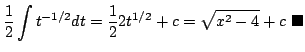 |