next up previous contents index
Next: 微分法(Differentiation Formulas) Up: 微分法(DIFFERENTIATION) Previous: 微分法(DIFFERENTIATION)   目次   索引

導関数(derivatives)

関数$ f(x)$とそのグラフ上の点 $ (x_{0},f(x_{0}))$が与えられたとき，どの直線を点 $ (x_{0},f(x_{0}))$における接線とよぶことができるでしょうか．

この問題に答えるために，小さな数$ h \neq 0$を選び，グラフ上に点 $ (x_{0} + h, f(x_{0} + h))$を印します．ここで，この2つの点を通る直線(割線(secant line))を引きます．この状況を記すと次のようになります．

図 2.1: 割線
\begin{figure}\begin{center} \includegraphics[width=11.6cm]{CALCFIG/secant.eps} \end{center}\vspace{-3em} \end{figure}
$ h$が徐々に右側から0に近づくと，割線は極限位置に近づいていきます．同様に$ h$が0に左側から近づくと，割線は右側から近づいたときと同じ極限位置に近づいていきます．この極限位置の直線をグラフ上の点 $ (x_{0},f(x_{0}))$における接線といいます．

割線の傾きは

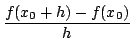
$\displaystyle \frac{f(x_{0} +h) - f(x_{0})}{h}$

で与えられます．これより，接線の傾きは

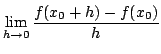
$\displaystyle \lim_{h \to 0}\frac{f(x_{0} +h) - f(x_{0})}{h}$

となります．

これが，図形を用いた接線の考え方です．

ここからは，このような極限値をもっと系統立てて学んでいきます．

定義 2..1  

関数 $ f(x)$ が $ x_{0}$ を含むある区間で定義されているとき，極限値

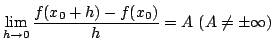
$\displaystyle \lim_{h \rightarrow 0} \frac{f(x_{0}+h) - f(x_{0})}{h} = A (A \neq \pm \infty) $

が存在するならば，関数 $ f(x)$ は， $ x = x_{0}$ で微分可能(differentiable) であるといいます．また，この極限値 $ A$ を点 $ x_{0}$ における微分係数といい， $ f^{\prime}(x_{0})$ で表わします．


例題 2..1  

$ f(x) = \sin{x}$ の微分係数 $ f^{\prime}(0)$ を求めてみましょう．

解

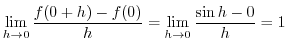
$\displaystyle \lim_{h \rightarrow 0} \frac{f(0+h) - f(0)}{h} = \lim_{h \rightarrow 0}\frac{\sin{h} - 0}{h} = 1 \ $

(例題1.3参照) したがって， $ f^{\prime}(0) = 1$ となります． $ \blacksquare$

これをグラフで見てみましょう．

図 2.2: 微分係数
\begin{figure}\begin{center} \includegraphics[width=6cm]{CALCFIG/Fig2-1-1.eps} \end{center}\vspace{-3em}%ce \end{figure}
図2.2を見て下さい．この図には $ \sin{x}$ のグラフとその接線(tangent line) $ y = x$ が描かれています．ここで， $ \sin{x}$ の $ x = 0$ での微分係数と接線 $ y = x$ の傾きが同じであることに気付いて下さい．つまり， $ f^{\prime}(0)$ は関数 $ f(x)$ の $ x = 0$ での接線の傾きを表わします．このことから，$ y = f(x)$のグラフ上の点 $ (x_{0},y_{0})$での接線の方程式は，

$\displaystyle y - y_{0} = f'(x_{0})(x - x_{0})$


となります．また，接線と垂直な線を法線といい，$ y = f(x)$のグラフ上の点 $ (x_{0},y_{0})$での法線の方程式は，接線と法線は垂直であることから，

$\displaystyle y - y_{0} = -\frac{1}{f'(x_{0})}(x - x_{0})$


となります．

例題 2..2  

$ f(x) = x^{2}$のグラフ上の点$ (-3,9)$における接線と法線の方程式を求めよ．

解 $ f'(x) = 2x$より，点$ (-3,9)$での接線の傾きは $ f'(-3) = -6$. したがって，求める接線の方程式は，

$\displaystyle y - 9 = -6(x - (-3))$

つまり，

$\displaystyle y - 9 = -6(x + 3)$

法線の方程式は，

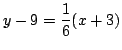
$\displaystyle y - 9 = \frac{1}{6}(x + 3)$

となります． $ \blacksquare$

$ f(x)$ が $ x_{0}$ で微分可能でなくても

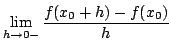
$\displaystyle \lim_{h \rightarrow 0-} \frac{f(x_{0}+h) - f(x_{0})}{h} $

または

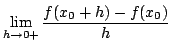
$\displaystyle \lim_{h \rightarrow 0+} \frac{f(x_{0}+h) - f(x_{0})}{h} $

が存在することがあります．その場合，最初の値を 左側微分係数(left-hand derivative ) といい， $ f_{-}^{\prime}(x_{0})$ で表わし，後の値を 右側微分係数(right-hand derivative) といい， $ f_{+}^{\prime}(x_{0})$ で表わします．微分可能の定義より， $ f_{-}^{\prime}(x_{0})$ と $ f_{+}^{\prime}(x_{0})$ が共に存在し，かつ両者が等しいときに限り $ f(x)$ は $ x = x_{0}$ で微分可能となります．

例題 2..3  

$ f(x) = \vert x\vert$ は $ x = 0$ で微分可能か調べてみましょう．

解 まず， $ f_{-}^{\prime}(0)$ を求めると

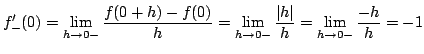
$\displaystyle f_{-}^{\prime}(0) = \lim_{h \rightarrow 0-}\frac{f(0+h) - f(0)}{h... ...ightarrow 0-}\frac{\vert h\vert}{h} = \lim_{h \rightarrow 0-}\frac{-h}{h} = -1 $

次に $ f_{+}^{\prime}(0)$ を求めると

$\displaystyle f_{+}^{\prime}(0) = \lim_{h \rightarrow 0+}\frac{f(0+h) - f(0)}{h... ...\rightarrow 0+}\frac{\vert h\vert}{h} = \lim_{h \rightarrow 0+}\frac{h}{h} = 1 $

となります．よって $ f(x) = \vert x\vert$ は $ x = 0$ で微分可能ではありません． $ \blacksquare$

$ f(x) = \vert x\vert$ は $ x = 0$ で微分可能ではありませんでしたが， $ x = 0$ で連続です．微分可能性と連続性の間にはどんな関係があるのでしょうか．

定理 2..1  

関数 $ f(x)$ が $ x = x_{0}$ で微分可能ならば， $ f(x)$ は $ x = x_{0}$ で連続である．


証明 $ \lim_{x \rightarrow x_{0}} f(x) = f(x_{0})$ を示せばよいでしょう．そこで

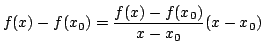
$\displaystyle f(x) - f(x_{0}) = \frac{f(x) - f(x_{0})}{x - x_{0}}(x - x_{0}) $

と書き直し， $ x$ を $ x_{0}$ に近づけると

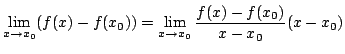
$\displaystyle \lim_{x \rightarrow x_{0}}(f(x) - f(x_{0})) = \lim_{x \rightarrow x_{0}}\frac{f(x) - f(x_{0})}{x - x_{0}}(x - x_{0})$

ここで $ f(x)$ は $ x = x_{0}$ で微分可能であることに注意すると
$\displaystyle \lim_{x \rightarrow x_{0}}(f(x) - f(x_{0}))$ $\displaystyle =$ $\displaystyle \lim_{x \rightarrow x_{0}}\frac{f(x) - f(x_{0})}{x - x_{0}}\cdot \lim_{x \rightarrow x_{0}}(x - x_{0})$  
  $\displaystyle =$ $\displaystyle f^{\prime}(x_{0}) \cdot \lim_{x \rightarrow x_{0}}(x - x_{0}) = 0$  

よって

$\displaystyle \lim_{x \rightarrow x_{0}} f(x) = f(x_{0}) \ensuremath{ \blacksquare}$

この逆，つまり連続ならば微分可能とはならないことは例題2.1 でみました．では関数が連続で微分可能ではないとき，グラフはどんな形をしているのでしょうか．図2.3を見てみましょう．$ f(x)$ が $ x = x_{0}$ で微分可能でないとき， $ f_{+}^{\prime}(x_{0}) \neq f_{-}^{\prime}(x_{0})$ となっています．これより $ f_{+}^{\prime}(x_{0})$ と $ f_{-}^{\prime}(x_{0})$ が存在し等しくないときには，関数 $ f(x)$ は $ x = x_{0}$ でとがっていることが分かります．

図 2.3: 微分可能?
\begin{figure}\begin{center} \includegraphics[width=6cm]{CALCFIG/Fig2-1-2.eps} \end{center}\end{figure}

関数 $ f(x)$ が，ある区間Iの各点で微分可能のとき $ f(x)$ は 区間Iで微分可能(differentiable on I) であるといいます．この場合，区間Iの各点にそこでの微分係数を対応させることにより定まる関数を $ f(x)$ の 導関数(derivative) といい，

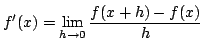
$\displaystyle f^{\prime}(x) = \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h} $

で表わします．ほかにも，

$\displaystyle \frac{df(x)}{dx}, Df(x) $

などの表わし方もあります．また，関数 $ f(x)$ の導関数を求めることを 微分する(differentiate) といいます．

例題 2..4  

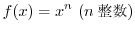$\displaystyle{f(x) = x^{n} \ (n \mbox{整数})}$ を微分してみましょう．

解

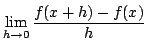$\displaystyle f^{\prime}(x)$ $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h}$  
  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{(x+h)^{n} - x^n}{h}$  
  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{nx^{n-1}h + \binom{n}{2}x^{n-2}h^2 + \cdots + h^n}{h} \ \ (2項定理)$  
  $\displaystyle =$ $\displaystyle nx^{n-1} + \lim_{h \rightarrow 0}[\binom{n}{2}x^{n-2}h + \binom{n}{3}x^{n-3}h^2 + \cdots + h^{n-1}] = nx^{n-1}$  

となります． $ \blacksquare$

$\displaystyle \frac{d}{dx}(x^{n}) = nx^{n-1}$


例題 2..5  

$ \displaystyle{f(x) = e^{x}}$ を微分してみましょう．

解

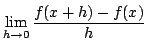$\displaystyle f^{\prime}(x)$ $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h}$  
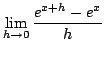  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{e^{x+h} - e^{x}}{h}$  
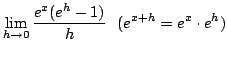  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{e^{x}(e^{h} - 1)}{h} (e^{x+h} = e^{x}\cdot e^{h})$  
  $\displaystyle =$ % latex2html id marker 65106 $\displaystyle e^{x}\lim_{h \rightarrow 0}\frac{e^{h} - 1}{h} = e^{x} (演習問題\ref{enshu:chouetsu}-1(b)参照)$  

となります． $ \blacksquare$

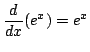
$\displaystyle \frac{d}{dx}(e^{x}) = e^{x}$


例題 2..6  

$ \displaystyle{f(x) = \sin{x}}$ を微分してみましょう．

解

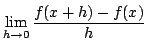$\displaystyle f^{\prime}(x)$ $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h}$  
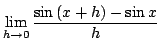  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{\sin{(x+h)} - \sin{x}}{h}$  
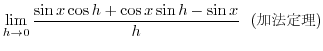  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{\sin{x}\cos{h} + \cos{x}\sin{h} - \sin{x}}{h} \ \ (加法定理)$  
  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}[\sin{x}\frac{\cos{h} - 1}{h} + \cos{x}\frac{\sin{h}}{h}]$  
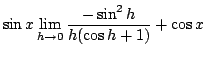  $\displaystyle =$ $\displaystyle \sin{x} \lim_{h \rightarrow 0}\frac{-\sin^{2}{h}}{h(\cos{h} + 1)} + \cos{x}$  
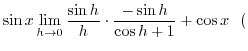  $\displaystyle =$ $\displaystyle \sin{x} \lim_{h \rightarrow 0}\frac{\sin{h}}{h}\cdot\frac{-\sin{h}}{\cos{h} + 1} + \cos{x} \ \ ($例題% latex2html id marker 65145 $\displaystyle \ref{limsin}$参照$\displaystyle ) $  
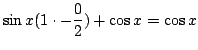  $\displaystyle =$ $\displaystyle \sin{x}(1 \cdot - \frac{0}{2}) + \cos{x} = \cos{x}$  

となります． $ \blacksquare$

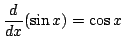
$\displaystyle \frac{d}{dx}(\sin{x}) = \cos{x}$


例題 2..7  

$ \displaystyle{f(x) = \cos{x}}$ を微分してみましょう．

解

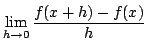$\displaystyle f^{\prime}(x)$ $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h}$  
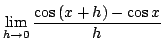  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{\cos{(x+h)} - \cos{x}}{h}$  
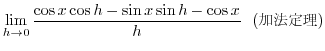  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{\cos{x}\cos{h} - \sin{x}\sin{h} - \cos{x}}{h} \ \ (加法定理)$  
  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}[\cos{x}\frac{\cos{h} - 1}{h} - \sin{x}\frac{\sin{h}}{h}]$  

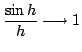ここで， $ \displaystyle{\frac{\cos{h} - 1}{h} \longrightarrow 0}$. また， $ \displaystyle{\frac{\sin{h}}{h} \longrightarrow 1}$より，

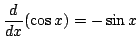
$\displaystyle \frac{d}{dx}(\cos{x}) = -\sin{x}$


$ \blacksquare$

関数の導関数を，定義に基づいて求めるのは容易ではないことが分かりました．そこで，導関数の計算に必要な公式をまとめておきます．

定理 2..2  

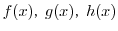[微分公式] $f(x)，g(x)，h(x)$ が微分可能のとき次式が成り立つ．

$ \displaystyle{(1) (f(x) \pm g(x))^{\prime} = f^{\prime}(x) \pm g^{\prime}(x) }$ (和の微分法)

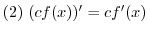$\displaystyle{(2) \ (cf(x))^{\prime} = cf^{\prime}(x)}$ (c : 定数)

$ \displaystyle{(3) (f(x)g(x))^{\prime} = f^{\prime}(x)g(x) + f(x)g^{\prime}(x)}$ (積の微分法)

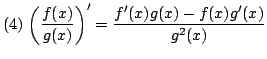$ (4) \displaystyle{\left(\frac{f(x)}{g(x)}\right)^{\prime} = \frac{f^{\prime}(x)g(x) - f(x)g^{\prime}(x)}{g^{2}(x)}} $ (商の微分法)


証明 $ (3)$ の証明．

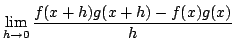$\displaystyle (f(x)g(x))^{\prime}$ $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{f(x+h)g(x+h) - f(x)g(x)}{h}$  
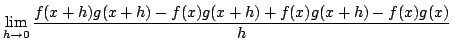  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{f(x+h)g(x+h) - f(x)g(x+h) + f(x)g(x+h) - f(x)g(x)}{h}$  
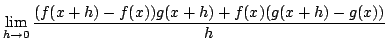  $\displaystyle =$ $\displaystyle \lim_{h \rightarrow 0}\frac{(f(x+h)- f(x))g(x+h) + f(x)(g(x+h) - g(x))}{h}$  
  $\displaystyle =$ $\displaystyle f^{\prime}(x)g(x) + f(x)g^{\prime}(x)$  

ほかの場合も同様にしてできます． $ \blacksquare$

例題 2..8  

微分公式を使って $ \displaystyle{y = 3x^{3} + 2x +3}$ の導関数を求めてみましょう．

解

$\displaystyle y^{\prime}$ $\displaystyle =$ $\displaystyle (3x^{3} + 2x +3)^{\prime}$  
  $\displaystyle \underbrace{=}_{和の微分法}$ $\displaystyle (3x^3)^{\prime} + (2x)^{\prime} + 3^{\prime}$  
  $\displaystyle =$ $\displaystyle 3\cdot 3x^2 + 2 = 9x^2 + 2$  

となります． $ \blacksquare$

例題 2..9  

微分公式を使って $ \tan{x}$ の導関数を求めてみましょう．

解

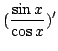$\displaystyle (\tan{x})^{\prime}$ $\displaystyle =$ $\displaystyle (\frac{\sin{x}}{\cos{x}})^{\prime}$  
  $\displaystyle \underbrace{=}_{商の微分法}$ $\displaystyle \frac{(\sin{x})^{\prime}\cos{x} - \sin{x}(\cos{x})^{\prime}}{\cos^{2}{x}}$  
  $\displaystyle =$ $\displaystyle \frac{\cos^{2}{x} + \sin^{2}{x}}{\cos^{2}{x}}$  
  $\displaystyle =$ $\displaystyle \frac{1}{\cos^{2}{x}} = \sec^{2}{x}$  

となります． $ \blacksquare$

$\displaystyle \frac{d}{dx}(\tan{x}) = \sec^{2}{x}$


図 2.4: 微分
\begin{figure}\begin{center} \includegraphics[width=6cm]{CALCFIG/Fig2-1-3.eps} \end{center}\vspace{-3em} \end{figure}

変数 $ x$ が，ある $ x$ から $ x+h$ まで変化するときの変動量$ h$ を $ x$ の 増分(increment) といい， $ \Delta x$ で表わし，これに対応する $ y$ の変動量 $ f(x+\Delta x) - f(x)$ を $ y$ の増分といい， $ \Delta y$ で表わすと， $ f^{\prime}(x)$ は次のように表わすことができます．

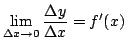
$\displaystyle \lim_{\Delta x \rightarrow 0}\frac{\Delta y}{\Delta x} = f^{\prime}(x) $

つまり，

$\displaystyle \Delta y = f^{\prime}(x) \Delta x + \circ(\Delta x) (\Delta x \rightarrow 0)$

ここで $ \circ(\Delta x)$ とは

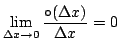
$\displaystyle \lim_{\Delta x \rightarrow 0}\frac{\circ(\Delta x)}{\Delta x} = 0 $

ということです．したがって， $ f^{\prime}(x)\Delta x$ が $ \Delta y$ の主要な部分とみなせます．そこで，これを点 $ x$ における関数 $ y = f(x)$ の 微分(differential) といい， $ dy$ または $ df(x)$ で表わします．つまり

$\displaystyle dy = df(x) = f^{\prime}(x){\Delta x} $

特に， $ f(x) = x$ のときは， $ f^{\prime}(x) = 1$ より

$\displaystyle df(x) = dx = \Delta x $

つまり，独立変数について増分と微分が一致します．これから

$\displaystyle dy = f^{\prime}(x)dx $

となり， $ dy,dx$ にそれぞれ別々に意味を持たせることができました．

例題 2..10  

$ \displaystyle{y = f(x) = \sin{x}}$ の微分を求めてみましょう．

解

$\displaystyle dy = f^{\prime}(x)dx = \cos{x}dx $

となります． $ \blacksquare$

確認問題


1.
次の関数の導関数を定義に基づいて求めよう．

(a) $ \displaystyle{f(x) = c}$ (b) $ \displaystyle{f(x) = \sqrt{x-1}}$ (c) $ \displaystyle{f(x) = \frac{1}{x^{2}}}$

2.
次の関数の$ x = 2$での微分係数を定義に基づいて求めよう．

(a) $ \displaystyle{f(x) = 5x - x^{2}}$ (b) $\displaystyle{f(x) = （3x - 7)^{2}}$

3.
次の曲線上の与えられた$ a$に対応する点における接線の方程式を求めよう．

(a) $ \displaystyle{f(x) = x^{2} - 5x + 3, a = 2}$ (b) $ \displaystyle{f(x) = 5 - x^{3}, a = 2}$ (c) $ \displaystyle{f(x) = \sqrt{x}, a = 4}$

4.
次の関数の導関数を求めよう．

(a) $ \displaystyle{y = 11x^{5} - 6x^{3} + 8}$ (b) $ \displaystyle{y = -\frac{1}{x^{2}}}$ (c) $ \displaystyle{y = (x^{2} - 1)(x-3)}$

(d) $ \displaystyle{y = \frac{x-1}{x-2}}$ (e) $ \displaystyle{y = \frac{x^{2}-1}{2x+3}}$ (f) $ \displaystyle{y = \frac{6 - 1/x}{x-2}}$ (g) $ \displaystyle{y = \frac{1 + x^{4}}{x^{2}}}$

演習問題


1.
次の関数の導関数を定義に基づいて求めよう．

(a) $ \displaystyle{f(x) = \cos{3x}}$ (b) $\displaystyle{f(x) = (x + 2)^{n} \ (n : \mbox{整数})}$

2.
次の関数の微分を求めよう．

(a) $ \displaystyle{f(x) = x^4}$ (b) $ \displaystyle{f(x) = e^{x}}$

3.
次の関数の $ x = 0$ における右側微分係数，および左側微分係数を求めよう．

(a) $ \displaystyle{f(x) = \vert x^2 + x\vert}$ (b) $ \displaystyle{f(x) = \left\{\begin{array}{ll} x^2 \sin{\frac{1}{x}}, & x \neq 0\\ 0, & x = 0 \end{array}\right.}$ (c) $ \displaystyle{f(x) = \sqrt{x^3 + x^2}}$

4.
次の関数の導関数を求めよう．

(a) $ \displaystyle{y = \frac{3x-1}{x^{2} + 1}}$ (b) $ \displaystyle{y = \sec{x}}$ (c) $ \displaystyle{y = {\rm cosec}{x}}$ (d) $ \displaystyle{y = \cot{x}}$

(e) $ \displaystyle{y = x^{2}e^{x}}$ (f) $ \displaystyle{y = e^{x}\sin{x}}$ (g) $ \displaystyle{y = \frac{e^{x}}{\sin{x}}}$

next up previous contents index
Next: 微分法(Differentiation Formulas) Up: 微分法(DIFFERENTIATION) Previous: 微分法(DIFFERENTIATION)   目次   索引