Bernoulli,Riccati's Differential Equations

Exercise 1.7

1. Solve the following differential equations.

(a) $\ xy^{\prime} - y = -y^{2}$
(b) $\ xy^{\prime} + y = y^{2}\log{x}$
(c) $\ y^{\prime} - y\cos{x} + y^{2}\cos{x} = 0$

2. Solve the following differential equations.

(a) $\ yy^{\prime} + y^{2} + 4x(x+1) = 0$
(b) $\ (y+1)y^{\prime} + x(y^{2} + 2y) = x$

3. Solve the following differential equations.
(a) $x^{2}y^{\prime} = -x^{2}y^{2} - 4xy -2$, Note that $f(x) = -2/x$ is a solution
(b) $xy^{\prime} = y - xy^{2} + x^{3}$

Answer
1. (a) Rewrite into the standard form.

$$y^{\prime} - \frac{1}{x} y = -\frac{1}{x}y^{2}$$

This is Bernoulli's equation. So, multiply $y^{-2}$ to the both sides.

$$y^{-2}y^{\prime} - \frac{1}{x} y^{1-2} = -\frac{1}{x}$$

Now we let $u = y^{1-2} = y^{-1}$. Then $u^{\prime} = -y^{-2}y^{\prime}$ implies that

$$- u^{\prime} - \frac{1}{x} u = - \frac{1}{x}$$

This is linear in $u$. Thus rewrite this into the standard form in $u$. Then

$$u^{\prime} + \frac{1}{x} u = \frac{1}{x}$$

Then we find $\mu$. Then $\mu = \exp(\int (1/x) dx) = \exp(\log{x}) = x$る. Multiply $\mu$ to the standard form. Then the left-hand side is the derivative of the product of $\mu$ and the dependent variable $u$.

$$( x u)^{\prime} = 1$$

Integrate both sides by $x$. Then

$$xu = \int 1 dx = x + c$$

Thus,

$$u = \frac{x + c}{x}$$

Substitute $u = y^{-1}$, we have

$$y = \frac{1}{u} = \frac{x}{x+c} \ \$$

(b) Rewrite into the standard form.

$$y^{\prime} + \frac{1}{x} y = \frac{1}{x}y^{2}\log{x}$$

This is Bernoulli's equation. Now multiply $y^{-2}$ to the both sides.

$$y^{-2}y^{\prime} + \frac{1}{x} y^{1-2} = \frac{\log{x}}{x}$$

Now let $u = y^{1-2} = y^{-1}$. Then $u^{\prime} = -y^{-2}y^{\prime}$ and

$$- u^{\prime} + \frac{1}{x} u = \frac{\log{x}}{x}$$

This is linear in $u$. Write this into the standard form in $u$.

$$u^{\prime} - \frac{1}{x} u = - \frac{\log{x}}{x}$$

Now we find $\mu$. Then $\mu = \exp(-\int (1/x) dx) = \exp(-\log{x}) = 1/x$. Multiply $u$ to the standard form. The left-hand side is the derivative of the product of $\mu$ and $u$. Then

$$( \frac{u}{x})^{\prime} = - \frac{\log{x}}{x^2}$$

Integrate both sides by $x$.

$$\frac{u}{x} = - \int \frac{\log{x}}{x^2} dx \ \left(\begin{array}{ll} v = \log{x} & dw = dx/x^2\\ dv = dx/x & w = - 1/x \end{array}\right )$$

$$= - [- \frac{\log{x}}{x} - \int \frac{-1}{x^2} dx ] = \frac{\log{x}}{x} + \frac{1}{x} + c$$

Therefore, $u = \log{x} + 1 + cx$ and

$$y = \frac{1}{u} = \frac{1}{\log{x} + 1 + cx} \ \$$

(c) Rewrite this into the standard form.

$$y^{\prime} - \cos{x} y = - y^2 \cos{x}$$

This is Bernoulli's equation. Then multiply $y^{-2}$ and simplify

$$y^{-2}y^{\prime} - \cos{x} y^{1-2} = - \cos{x}$$

Now let $u = y^{1-2} = y^{-1}$. Then we have $u^{\prime} = -y^{-2}y^{\prime}$ and

$$- u^{\prime} -\cos{x} u = - \cos{x}$$

This is a linear differential equation in $u$. Now write inot the standard form in $u$.

$$u^{\prime} + \cos{x} u = \cos{x}$$

Now we find the integrating factor $\mu$. Note that $\mu = \exp(\int \cos{x} dx) = \exp(\sin{x}) = e^{\sin{x}}$. Multiply $\mu$ to the standard form. Then the left-hand side is the derivative of the product of $\mu$ and $u$.

$$( e^{\sin{x}} u)^{\prime} = e^{\sin{x}} \cos{x}$$

Integrate both sides by $x$. We have

$$e^{\sin{x}} u = \int e^{\sin{x}} \cos{x} dx \ \left(\begin{array}{ll} t = \sin{x} & dt = \cos{x}dx \end{array}\right )$$

$$= \int e^{t} dt = e^{t} + c = e^{\sin{x}} + c$$

Therefore, $u = (e^{\sin{x}} + c)/e^{\sin{x}}$ and

$$y = \frac{1}{u} = \frac{e^{\sin{x}} }{e^{\sin{x}} + c } \ \$$

2. (a) Rewrite into the standard form.

$$y^{\prime} + y = - 4x(x+1) y^{-1}$$

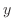This is Bernoulli's equataion. Now multiply $y$ to both sides.

$$yy^{\prime} + y^2 = - 4x(x+1)$$

Now let $u = y^{2}$. Then $u^{\prime} = 2yy^{\prime}$ and

$$\frac{ u^{\prime}}{2} + u = - 4x(x+1)$$

This is linear in $u$. So, write into the standard form in $u$.

$$u^{\prime} + 2 u = - 8x(x+1)$$

Now we find the integrating factor $\mu$. Note that $\mu = \exp(\int 2 dx) = \exp(2x) = e^{2x}$. Then multiply $u$ to the standard form，The left-hand side is the derivative of the product of $\mu$ and $u$.

$$( e^{2x} u)^{\prime} = - 8x(x+1)e^{2x}$$

Integrate both sides by $x$.

$$e^{2x} u = - 8\int (x^{2}e^{2x} + xe^{2x}) dx \ \left(\begin{array}{ll} v = x^{2} & dw = e^{2x}dx\\ dv = 2x dx & w = \frac{1}{2}e^{2x} \end{array}\right )$$

$$= - 8(\frac{1}{2}x^{2}e^{2x} - \int x e^{2x} dx + \int xe^{2x} dx + c)$$

$$= - 4 x^{2}e^{2x} + c$$

Therefore,

$$y^{2} = u = - 4 x^{2} + ce^{-2x} \ \$$

(b) In $\ (y+1)y^{\prime} + x(y^{2} + 2y) = x$，we let $u = y^{2} + 2y$. Then $u^{\prime} = 2yy^{\prime} + 2y^{\prime} = 2y^{\prime}(y+1)$. Now put this back into the original equation.

$$\frac{u^{\prime}}{2} + ux = x$$

This is linear in $u$. So, write this into the standard form in $u$.

$$u^{\prime} + 2x u = 2x$$

Now we find $\mu$. Then $\mu = \exp(\int 2x dx) = \exp(x^{2}) = e^{x^{2}}$. Then multiply this to the standard form. Then the left-hand side is the derivative of the product of $\mu$ and $u$.

$$( e^{x^{2}} u)^{\prime} = 2x e^{x^{2}}$$

Integrate both sides by $x$.

$$e^{x^{2}} u = 2 \int x e^{x^{2}} dx \ \left(\begin{array}{ll} t = x^{2} & dt = 2x dx \end{array}\right )$$

$$= \int e^{t} dt = e^{t} + c = e^{x^{2}} + c$$

Thereforem $u = 1 + ce^{-x^{2}}$ and

$$u = y^{2} + 2y = 1 + ce^{-x^{2}} \ \$$

3. (a) Rewrite this into the standard form.

$$y^{\prime} = - y^{2} - \frac{4x}{x^{2}}y - \frac{2}{x^{2}}$$

This is Riccati's equation. Since $f(x) = -2/x$ is a solution of the above differential equation, let $y = -2/x + 1/u$. Then $y^{\prime} = \frac{2}{x^{2}} - u^{\prime}/u^{2}$. Write this into the standard form.

$$\frac{2}{x^{2}} - u^{\prime}/u^{2} = - ( -\frac{2}{x} + \frac{1}{u})^{2} - \frac{4x}{x^{2}}(-\frac{2}{x} + \frac{1}{u}) - \frac{2}{x^{2}}$$

$$= - \frac{4}{x^2} + \frac{4}{xu} - \frac{1}{u^{2}} + \frac{8}{x^{2}} - \frac{4}{xu} - \frac{2}{x^{2}}$$

$$= \frac{2}{x^{2}} - \frac{1}{u^{2}}$$

Simplifing, we have $u^{\prime} = 1$. Therefore,

$$u = x + c$$

Here, since $y = -2/x + 1/u$, we have

$$y = -\frac{2}{x} + \frac{1}{x + c} = -\frac{x + 2c}{x(x+c) } \ \$$

(b) Rewrite this into the standard form

$$y^{\prime} = - y^{2} + \frac{1}{x}y + x^2$$

This is Riccati's equation. Then we find a solution of this equation. Note that $f(x) = x$ is a solution of this equation. We let $y = x + 1/u$. Then $y^{\prime} = 1 - u^{\prime}/u^{2}$. Put these back into the standard form.

$$1 - u^{\prime}/u^{2} = - ( x + \frac{1}{u})^{2} + \frac{1}{x}(x + \frac{1}{u}) + x^{2}$$

$$= - x^{2} - 2\frac{x}{u} - \frac{1}{u^{2}} + 1 + \frac{1}{ux} + x^{2}$$

$$= 1 - 2\frac{x}{u} + \frac{1}{ux} - \frac{1}{u^2}$$

Simplyfying to get $u^{\prime} = 2ux - \frac{u}{x} + 1$. This is a linear differential equation. So, write in the standard form.

$$u^{\prime} + ( \frac{1}{x} - 2x)u = 1$$

Now finding the integrating factir $\mu$, we have $\mu = \exp(\int (1/x - 2x) dx) = \exp(\log{x} - x^{2}) = e^{\log{x}}e^{-x^{2}} = xe^{-x^{2}}$. Multiplying $\mu$ to the standard form in $u$. Then the left-hand side is the derivative of the product of $\mu$ and $u$. Thus,

$$( xe^{-x^{2}} u)^{\prime} = x e^{-x^{2}}$$

Integrate both sides with respect to $x$.

$$xe^{x^{2}} u = \int x e^{x^{2}} dx \ \left(\begin{array}{ll} t = -x^{2} & dt = -2x dx \end{array}\right )$$

$$= -\frac{1}{2} \int e^{t} dt = -\frac{1}{2} e^{t} + c = -\frac{1}{2}e^{- x^{2}} + c$$

Therefore, $u = -1/2x + c/xe^{-x^{2}} = ( 2ce^{x^2} - 1) /2x$. Hence,

$$y = x + \frac{1}{u} = x + \frac{2x}{2ce^{x^2} - 1} \ \$$