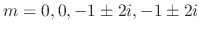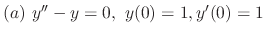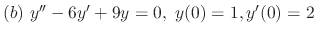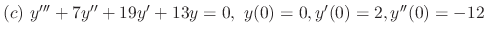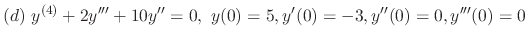Next: Method of undetermined coefficients Up: Higher order homogeneous linear Previous: Higher order homogeneous linear Contents Index
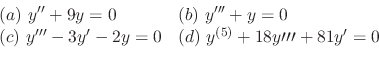
 The roots of the characteristic equation of the 4th-order linear homogeneous differential equation are
The roots of the characteristic equation of the 4th-order linear homogeneous differential equation are
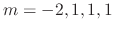
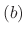 The roots of the characteristic equation of the 6th-order linear homogeneous differential equation are
The roots of the characteristic equation of the 6th-order linear homogeneous differential equation are