2.4 Properties of functions

1.

(a) $\displaystyle{-\frac{1}{3}}$ (b) $\displaystyle{\sqrt{1 - \left(\frac{2}{\pi}\right)^2}}$ (c) $e - 1$

2.

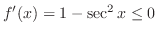$f'(x) = 1 - \sec^{2}{x} \leq 0$ and the equality holds only for 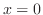$x = 0$ ． 3.

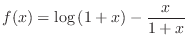(a) Let $\displaystyle{f(x) = \log{(1 + x)} - \frac{x}{1+x}}$ . Then we have $f(0) = 0$ . Thus for $x > 0$ , we only need to show $f'(x) > 0$ ．

$\displaystyle f'(x) = \frac{1}{1+x} - \frac{1}{(1+x)^2} = \frac{x}{(1+x)^2} > 0$

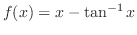(b) Let $\displaystyle{f(x) = x - \tan^{-1}{x}}$ . Then $f(0) = 0$ . Thus for $x > 0$ , we only need to show $f'(x) > 0$ ．

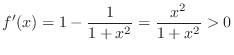
$\displaystyle f'(x) = 1 - \frac{1}{1+x^2} = \frac{x^2}{1+x^2} > 0$

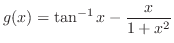Next, let $\displaystyle{g(x) = \tan^{-1}{x} - \frac{x}{1+x^2}}$ . Then， $f(0) = 0$

$f(0) = 0$

. Thus for $x > 0$

$x > 0$

, we need to show $f'(x) > 0$

$f'(x) > 0$

．

$\displaystyle f'(x) = \frac{1}{1+x^2} - \frac{1 - x^2}{(1+x^2)^2} = \frac{2x^2}{(1+x^2)^2} > 0$

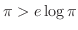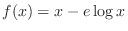(c) Take logarithm to both sides，show $\pi > e\log{\pi}$ ．Let $f(x) = x - e\log{x}$ . Then $f(e) = 0$ ．For $x > e$

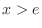
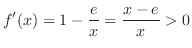
$\displaystyle f'(x) = 1 - \frac{e}{x} = \frac{x - e}{x} > 0$

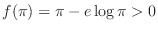
Thus $f(\pi) = \pi - e\log{\pi} > 0$

4.

(a) local maximum 7 at 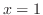$x = 1$ ，local minimum 3 at $x = 3$

(b) local minimum 0 at 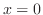$x = 0$ ， local maximum 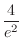$\displaystyle{\frac{4}{e^2}}$ at $x = 2$

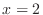
$\begin{figure}\par \begin{center}\includegraphics[width=8cm]{CALCFIG/Fig9-2-6-3.eps}\end{center}\par \end{figure}$