Next: Total Differential and Tangent Up: Partial Differentiation Previous: Limit of Functions of Contents Index
| Understanding |
|---|
A partial derivative of  at at  with respect to with respect to  is a curve shows up by slicing the surface given by is a curve shows up by slicing the surface given by
 by the plane by the plane 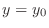 . Thus differentiate . Thus differentiate
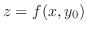 by by  gives the answer. gives the answer.
|
Consider the limit of a function
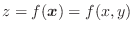 at point
at point
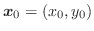 . Let
. Let 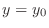 be a constant and a function
be a constant and a function
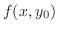 of
of  is differentiable at
is differentiable at 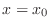 . Then
. Then
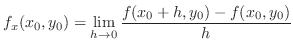
 .
Similarly, Keep
.
Similarly, Keep 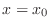 a constant and a function
a constant and a function 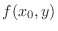 of
of  is differentiable at
is differentiable at 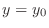 . Then
. Then
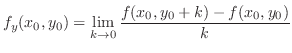
 .
.
NOTE If
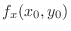 exists, then
exists, then  is called partially differentiable
with respect
is called partially differentiable
with respect  at
at  . If
. If  is differentiable at esch
is differentiable at esch  in
in 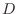 , then
, then  is called partially differentiable on
is called partially differentiable on 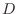 with respect
with respect  .
.
| Partially Differentiable |
|---|
 is differentiable with respect to is differentiable with respect to  at at  if and only if if and only if 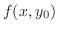 is differentiable at is differentiable at 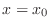 . Similarly, . Similarly,  is differentiable with respect to is differentiable with respect to  at at  if and only if if and only if 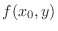 is differentiable at is differentiable at 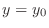 . .
|
 .
.
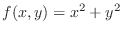
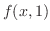 is a curve given by slicing the surface
is a curve given by slicing the surface
 with the plane
with the plane  . Then
. Then
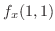 is a derivative of the curve
is a derivative of the curve
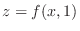 with respect to
with respect to  at
at 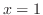 .
.
SOLUTION To slice the surface
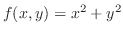 by the plane
by the plane  , it is enough to consider
, it is enough to consider
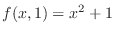 . Now differentiate
. Now differentiate 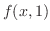 by
by  . Then we have
. Then we have
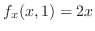 . Thus,
. Thus,
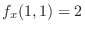

 .
.
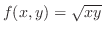
SOLUTION
Let 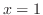 . Then slice the surface
. Then slice the surface
 by the plane
by the plane 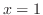 to get the curve
to get the curve
 . Now differentiate
. Now differentiate 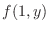 with respect to
with respect to  to obtain
to obtain
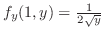 . Thus,
. Thus,
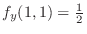

A partial derivative of  with respect to
with respect to  is the derivative of
is the derivative of  by keeping
by keeping  as constant. More precisely,
as constant. More precisely,
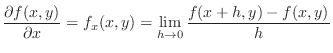
 with respect to
with respect to  is
is
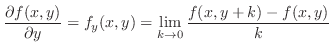
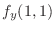 is the derivative
is the derivative 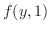 with respect to
with respect to  at
at  .
.
| Understanding |
|---|
To find the partial derivative of  with respect to with respect to  , fix , fix  and differentiate and differentiate  by by  . . 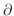 is read del, dee, partial. is read del, dee, partial.
|
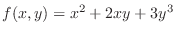 2.
2.
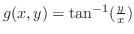
Note that to find the partial derivative of  with respect to
with respect to  , we treat
, we treat  as constant. Thus
as constant. Thus
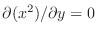 .
.
SOLUTION
1. To find
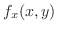 , treat
, treat  as constant and differentiate
as constant and differentiate  with respect ot
with respect ot  .
.
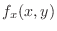 |
 |
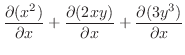 |
|
 |
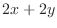 |
 as constant and differentiate with respect to
as constant and differentiate with respect to 
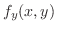 |
 |
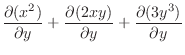 |
|
 |
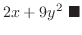 |
| Review |
|---|
1.
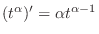
2. 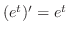
3. 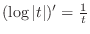
4. 
5. 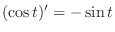
6. 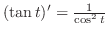
7. 
8. 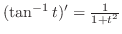
|
2.
Let
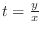 . Then
. Then
 |
 |
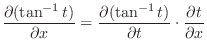 |
|
 |
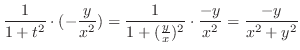 |
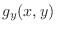 |
 |
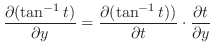 |
|
 |
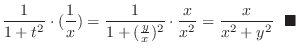 |
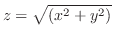 2.
2.
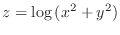
SOLUTION
1. Let
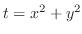 . Then
. Then
 |
 |
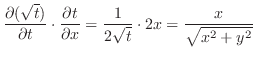 |
|
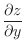 |
 |
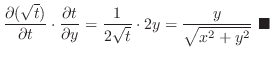 |

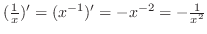
2. Let
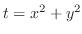 . Then
. Then
 |
 |
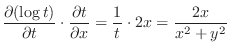 |
|
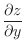 |
 |
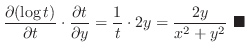 |
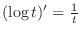
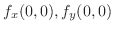 . Then determine the following function is partially differentiable with respect to
. Then determine the following function is partially differentiable with respect to 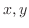 or not.
or not.
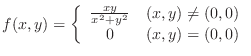
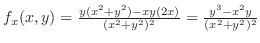 . Now substitute
. Now substitute  to get
to get
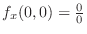 . Then conclude that
. Then conclude that  is not partially differentiable is not right. The reason is that
is not partially differentiable is not right. The reason is that
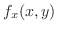 is not continuous at
is not continuous at  . Thus substituting
. Thus substituting  is not allowed.
is not allowed.
SOLUTION To find
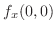 , substitute
, substitute 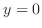 and find
and find 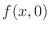 . Then
. Then
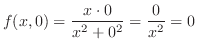
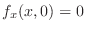 . Thus,
. Thus,
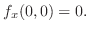
Since
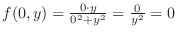 , we have
, we have
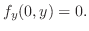 Thus
Thus
 .
.
Therefore,  is partially differentiable with respect to
is partially differentiable with respect to 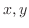 at
at 

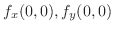 . Then determine the following function is partially differentiable with respect to
. Then determine the following function is partially differentiable with respect to 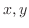 or not.
or not.
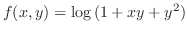
To find the function  is differentiable with respect to
is differentiable with respect to  at
at  , it is enough to check
, it is enough to check 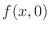 is differentiable with respect to
is differentiable with respect to  .
.
SOLUTION Since
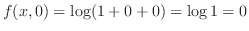 ,
,
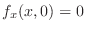 and
and
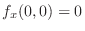 .
.
Note that
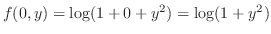 . Thus
. Thus
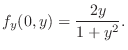
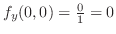 . Thus
. Thus  is partially differentiable with respect to
is partially differentiable with respect to  and
and  at
at 

|
Exercise
|
|
Exercise B
|