Area

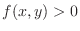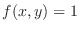For $f(x,y) > 0$ , the double integral $\iint_{\Omega}f(x,y)dxdy$ represents the volume of a solid whose base area is $\Omega$ and the height is $f(x,y)$ . If $f(x,y) = 1$ , then the double integral 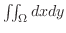$\iint_{\Omega}dxdy$ can be thought of the volume of solid whose base area is $\Omega$ and the height is 1. Now ignore the unit, then we can think of the area of $\Omega$ . Thus

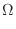$\displaystyle \Omega$ base area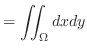 $\displaystyle = \iint_{\Omega}dxdy$

Area by Double Integral
If the region is given by the cartesian coordinate, then the area of the region can be evaluated without using double integrals. For the region bounded by the curves given by the polar coordinates, it is much easier to use double integral.

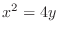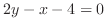
Example 5..6 Find the area of the region $\Omega$ bounded by the curve $\displaystyle{x^2 = 4y}$ and the line $\displaystyle{2y - x - 4 = 0}$

SOLUTION First find the intersection of two curves. Let 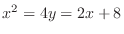$x^2 = 4y = 2x + 8$ . Then 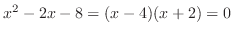$x^2 - 2x - 8 = (x-4)(x+2) = 0$ which implies $x = -2,4$ .

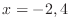$% latex2html id marker 29445 \includegraphics[width=4.5cm]{SOFTFIG-5/reidai5-6.eps}$

Now using the vertical simple, we have 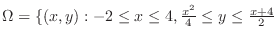$\Omega = \{(x,y): -2 \leq x \leq 4, \frac{x^2}{4} \leq y \leq \frac{x+4}{2}$ . Then

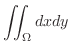$\displaystyle \iint_{\Omega}dxdy$	$\displaystyle =$	$\displaystyle \int_{-2}^{4}dx\int_{\frac{x^2}{4}}^{\frac{x+4}{2}}dy = \int_{-2}^{4}[\frac{x+4}{2} - \frac{x^2}{4}]dx$
	$\displaystyle =$	$\displaystyle \left[\frac{x^2}{4} +2x - \frac{x^3}{12}\right ]_{-2}^{4} = \frac{16 - 4}{4} + 2(4+2) -\frac{1}{12}(64 + 8)$
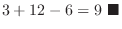	$\displaystyle =$	$\displaystyle 3 + 12 - 6 = 9 \ensuremath{ \blacksquare}$

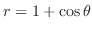Exercise 5..6 Find the area of the region $\Omega$ that lies inside the cardioid $\displaystyle{r = 1 + \cos{\theta}}$ but outside the circle $r = 1$

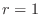Exercise5-6
$% latex2html id marker 29474 \includegraphics[width=3.5cm]{SOFTFIG-5/enshu5-6.eps}$

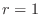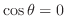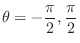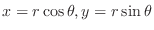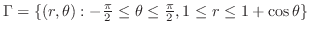SOLUTION First find the intersection of $r = 1 + \cos{\theta}$ and $r = 1$ . Then since $\cos{\theta} = 0$ , $\displaystyle{\theta = -\frac{\pi}{2}, \frac{\pi}{2}}$ . Thus by change of variables, $x = r\cos{\theta}, y = r\sin{\theta}$ , the region $\Omega$ is transformed into the region $\Gamma = \{(r,\theta) : -\frac{\pi}{2} \leq \theta \leq \frac{\pi}{2}, 1 \leq r \leq 1+\cos{\theta} \}$ . Thus

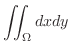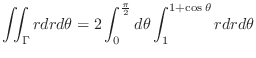$\displaystyle \iint_{\Omega}dxdy$	$\displaystyle =$	$\displaystyle \iint_{\Gamma}rdrd\theta = 2\int_{0}^{\frac{\pi}{2}}d\theta \int_{1}^{1+\cos{\theta}}rdrd\theta$
	$\displaystyle =$	$\displaystyle 2\int_{0}^{\frac{\pi}{2}} \left[\frac{r^2}{2}\right ]_{1}^{1+\cos... ...ta = 2\int_{0}^{\frac{\pi}{2}}\frac{2\cos{\theta} + \cos^{2}{\theta}}{2}d\theta$
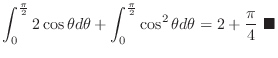	$\displaystyle =$	$\displaystyle \int_{0}^{\frac{\pi}{2}} 2\cos{\theta}d{\theta} + \int_{0}^{\frac... ...}{2}} \cos^{2}{\theta}d{\theta} = 2 + \frac{\pi}{4} \ensuremath{ \blacksquare}$

Check
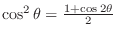
$\cos{2\theta} = 2\cos^{2}{\theta} - 1$ implies $\cos^{2}{\theta} = \frac{1 + \cos{2\theta}}{2}$ $\int_0^{\pi/2}\cos^{2}{\theta}\:d\theta = \int_0^{\pi/2}\frac{1+\cos{2\theta}}{... ...}{2}\sin{2\theta}\right]_0^{\pi/2} = \frac{1}{2}(\frac{\pi}{2}) = \frac{\pi}{4}$