Next: Integration of Rational Functions Up: Integration Previous: Integration by Substitution Contents Index
Integration by Parts
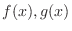 be differentiable functions. Then.
be differentiable functions. Then.

Understanding If you can not integrate by substitution, then use integration by parts.
NOTE
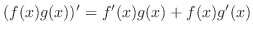 . Then
. Then
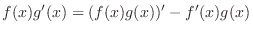 . Now integrate both sides with respect
. Now integrate both sides with respect  .
.

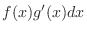 is hard to integrate, Rewrite by parts to get integration of
is hard to integrate, Rewrite by parts to get integration of
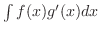 .
.
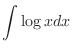 2.
2.
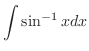
Integration by Parts If integrand contains a function such as
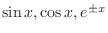 , then let
, then let 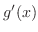 be one of these functions.
be one of these functions.
The integrand does not contain
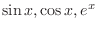 , let
, let
 .
SOLUTION 1.
.
SOLUTION 1.

Thus
When you use the integration by parts to get 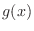 from
from 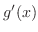 , you can ignore the constant.
, you can ignore the constant.
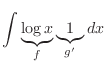 |
 |
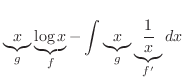 |
|
 |
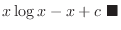 |
The integrand does not contain
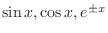 . Let
. Let
 .
2.
.
2.
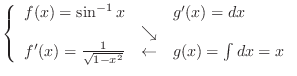
Thus

Now let
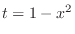 . Then
. Then
| Check |
|---|
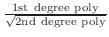 . .
|
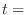 2nd degree poly
2nd degree poly
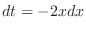 . Thus
. Thus
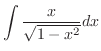 |
 |
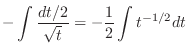 |
|
 |
 |

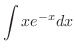 2.
2.
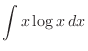 3.
3.
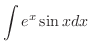
Integrand contains one of
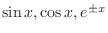 , let
, let
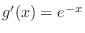 .
.
SOLUTION 1.
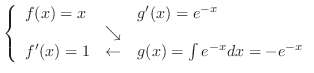
Thus,
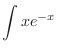 |
 |
 |
|
 |
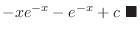 |
2.
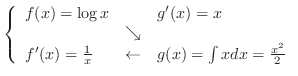
Thus,
 |
 |
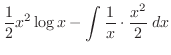 |
|
 |
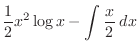 |
||
 |
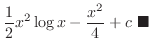 |
Integtand does not contain one of
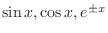 . Let
. Let
 .
.
3. Integrand contains two of
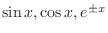 . Let
. Let 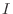 be the antiderivative.
be the antiderivative.
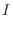 |
 |
 |
|
 |
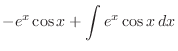 |
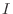 . So, we integrate again. .
. So, we integrate again. .
| Check |
|---|
Once you set  . Then use . Then use  again. again.
|
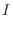 |
 |
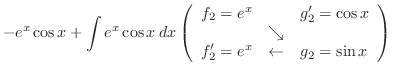 |
|
 |
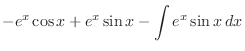 |
||
 |
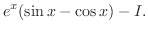 |
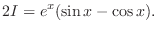

|
Exercise A
|
|
Exercise B
|