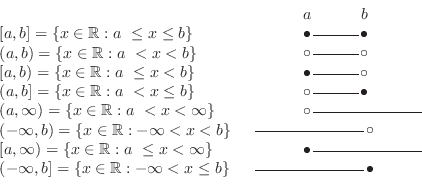Next: Inequalities Up: Introduction Previous: Introduction Contents Index
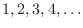 . Integers are the natural numbers with 0 and negative numbers such as
. Integers are the natural numbers with 0 and negative numbers such as
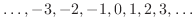 . Rational numbers are fractions of integers
. Rational numbers are fractions of integers
 with non-zero denominator
with non-zero denominator  . Irrational number are numbers with non repeated infinite decimals such as
. Irrational number are numbers with non repeated infinite decimals such as
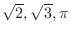 . Real numbers are either rational numbers or irrational numbers. Furthermore,
. Real numbers are either rational numbers or irrational numbers. Furthermore, 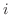 satisfying
satisfying  is called the imaginary unit.
is called the imaginary unit.
Symbols
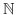 : Natural numbers
: Natural numbers
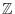 : Integers
: Integers
 : Rational numbers
: Rational numbers
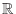 : Real numbers
: Real numbers
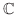 : Complex numbers
: Complex numbers
Complex numbers 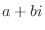 with the imaginary unit
with the imaginary unit 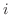 and real numbers
and real numbers 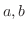 is called complex number.
is called complex number.
The set of real numbers can be expressed either using the symbol
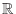
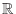 or the interval
or the interval
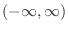 .
.
The real numbers can be thought of as points on a number line. In other words, every real number can be put into one-on-one correspondence with the point on the number line.
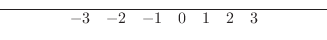
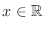 , we define
, we define
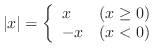
We call 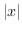 absolute value of
absolute value of  .
For example,
.
For example,
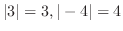 . Now carefully look at
. Now carefully look at
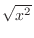 . For if
. For if  , then we have
, then we have
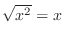 . For if
. For if 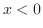 , then we have
, then we have
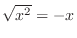 . This means that
. This means that
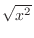 and
and 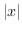 give rise the same number. Thus, we can say
give rise the same number. Thus, we can say
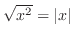 .
For example,
.
For example,
 .
Element of the set
A distinct object
.
Element of the set
A distinct object 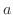 belongs to the set
belongs to the set 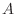 is called the element of the set
is called the element of the set 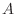 and denoted by
and denoted by 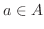 .
.
NOTE The set of natural numbers is
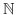 . Then we write
. Then we write
 and say 3 is an element of
and say 3 is an element of
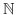 . 0 is not a natural number. Then we write
. 0 is not a natural number. Then we write
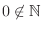 .
Subset
If all elements of
.
Subset
If all elements of 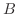 are also elments of
are also elments of 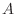 , then
, then 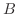 is subset of
is subset of 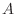 and denoted by
and denoted by
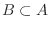 .
.
NOTE For
 , we must have
, we must have
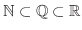 .
.
Union
The Union of tow sets 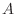 and
and 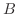 is the collection of points which are in
is the collection of points which are in 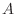 or in
or in 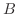 or in
or in 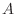 and
and 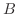 , and denoted by
, and denoted by 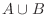 .
.
NOTE The set 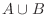 consists of all elements of
consists of all elements of 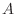 and elements of
and elements of 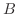 .
.
Intersection
The Intersection of two sets 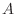 and
and 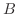 is the collection of points which are in
is the collection of points which are in 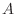 and
and 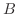 and denoted by
and denoted by 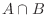 .
.
NOTE The set 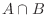 consists of elements which have both properties of
consists of elements which have both properties of 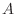 and
and 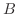 .
.
Subsets of