Inverse Trigonometric Functions

In this section, we study the inverse of trigonometric function.

Arcsin Let $\displaystyle{[-\frac{\pi}{2},\frac{\pi}{2}]}$ be the domain of the function $y = \sin{x}$ . Then the function $y = \sin{x}$ becomes one-to-one. Thus we can think of the inverse function. We write this function as

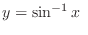$\displaystyle y = \sin^{-1}{x}$ or $\displaystyle y = \arcsin{x}$

and call arcsin x.

How to read $\sin^{-1}{x}$ $\sin^{-1}{x}$ is called arc sine of $x$ .

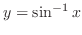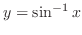NOTE The domain of $y = \sin^{-1}{x}$ is the range of $y = \sin{x}$ . Thus we have $[-1,1]$ . On the other hand, the range of $y = \sin^{-1}{x}$ is the domain of $y = \sin{x}$ . Thus we have $\displaystyle{[-\frac{\pi}{2},\frac{\pi}{2}]}$ . In other words,

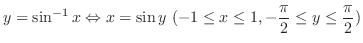
$\displaystyle y = \sin^{-1}{x} \Leftrightarrow x = \sin{y} (-1 \leq x \leq 1, -\frac{\pi}{2} \leq y \leq \frac{\pi}{2})$

In this situation, the value in this range $[-\frac{\pi}{2},\frac{\pi}{2}]$ is called principal value of arcsin $x$

Principal value There are many intervals satisfying 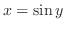$x = \sin{y}$ . Among many intervals, $\displaystyle{[-\frac{\pi}{2},\frac{\pi}{2}]}$ is chosen as most important interval. This is why the value in the range $[-\frac{\pi}{2},\frac{\pi}{2}]$ is called the principal value.

**Figure 1.34:** arcsin x
$\includegraphics[width=8cm]{SOFTFIG-1/Fig1-2-3.eps}$

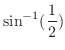
Example 1..11 Find the value of $\displaystyle{\sin^{-1}(\frac{1}{2})}$

**Figure 1.35:** Example1-11
$% latex2html id marker 38317 \includegraphics[width=3cm]{SOFTFIG-1/reidai1-11_gr4.eps}$

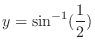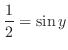SOLUTION Note that $\displaystyle{y = \sin^{-1}(\frac{1}{2})}$ means $\displaystyle{\frac{1}{2} = \sin{y}}$ . Note also the values of $y$ must be in the interval $\displaystyle{[\frac{-\pi}{2}, \frac{\pi}{2}]}$ .

What is the princial value Finding the trig inverse, make sure the principal value.

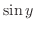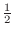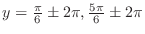Since $\sin{y}$ takes the value $\frac{1}{2}$ for $y = \frac{\pi}{6}\pm 2\pi,\frac{5\pi}{6} \pm 2\pi$ , $y$ must be in $\displaystyle{[\frac{-\pi}{2}, \frac{\pi}{2}]}$ . Thus we have $\displaystyle{y = \frac{\pi}{6}}$ $\blacksquare$

Exercise 1..11 Find the value of $\displaystyle{\sin^{-1}{(-\frac{1}{2})}}$ .

SOLUTION 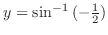$y = \sin^{-1}{(-\frac{1}{2})}$ is equivalent to 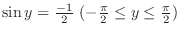$\sin y = \frac{-1}{2} (-\frac{\pi}{2} \leq y \leq \frac{\pi}{2})$ . Thus, $y = -\frac{\pi}{4}$ $\blacksquare$

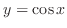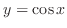Arccos Let the interval $[0,\pi]$ be the domain of $y = \cos{x}$ . Then $y = \cos{x}$ becomes one-to-one. Thus we can think of the inverse function. We write this function as

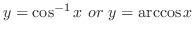
$\displaystyle y = \cos^{-1}{x} or y = \arccos{x}$

and call this function arccos $x$

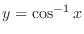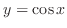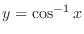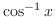NOTE The domain of the function $y = \cos^{-1}{x}$ is $[-1,1]$ which is the range of the function $y = \cos{x}$ . The range of the function $y = \cos^{-1}{x}$ is $[0,\pi]$ . Principal value of $\cos^{-1}{x}$ Note that 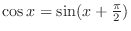$\cos{x} = \sin(x+\frac{\pi}{2})$ . Then the principal value of 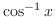$\cos^{-1}{x}$ is the principal value of 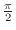$\sin^{-1}{x}$ + $\frac{\pi}{2}$ . Thus

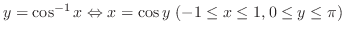
$\displaystyle y = \cos^{-1}{x} \Leftrightarrow x = \cos{y} (-1 \leq x \leq 1, 0 \leq y \leq \pi)$

The value in the interval $[0,\pi]$ is called pv of arccos $x$

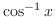**Figure:** Graph of $\cos^{-1}{x}$
$\includegraphics[width=3.5cm]{SOFTFIG-1/Fig1-2-4.eps}$

$\cos^{-1}{x}$ is called arc cosine of x.

Example 1..12 Find the value of $\displaystyle{\sin{\left(\cos^{-1}{\frac{1}{2}}\right)}}$ .

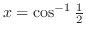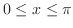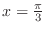SOLUTION Since $x = \cos^{-1}{\frac{1}{2}}$ is equivalent to $\cos x = \frac{1}{2}$ for $0 \leq x \leq \pi$ , we have $x = \frac{\pi}{3}$ . Thus

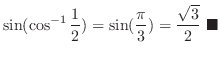
$\displaystyle \sin(\cos^{-1}\frac{1}{2}) = \sin(\frac{\pi}{3}) = \frac{\sqrt{3}}{2}\ensuremath{ \blacksquare}$

Exercise 1..12 Find the value $x$

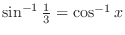which satisafies $\sin^{-1}\frac{1}{3} = \cos^{-1}{x}$ .

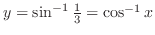SOLUTION Let $y = \sin^{-1}{\frac{1}{3}} = \cos^{-1}{x}$ . Then

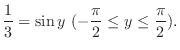
$\displaystyle \frac{1}{3} = \sin{y} (-\frac{\pi}{2} \leq y \leq \frac{\pi}{2}).$

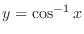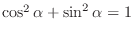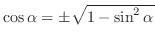Since $y = \cos^{-1}{x}$ , we have $x = \cos{y} \geq 0 (0 \leq y \leq \pi)$ . Note that, $\cos^{2}{\alpha} + \sin^{2}{\alpha} = 1$ can be written as $\cos{\alpha} = \pm \sqrt{1 - \sin^{2}{\alpha}}$ .

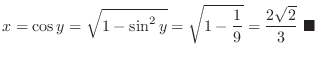
$\displaystyle x = \cos{y} = \sqrt{1 - \sin^{2}{y}} = \sqrt{1 - \frac{1}{9}} = \frac{2\sqrt{2}}{3}\ensuremath{ \blacksquare}$

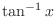**Figure:** Grph of $\tan^{-1}{x}$
$\includegraphics[width=3.5cm]{SOFTFIG-1/Fig1-2-5.eps}$

$\tan^{-1}{x}$ is called arc tangent of x.

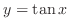ArcTan Let the interval $\displaystyle{[-\frac{\pi}{2},\frac{\pi}{2}]}$ be the domain of the function $y = \tan{x}$ . Then the function 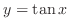$y = \tan{x}$ becomes one-to-one. Thus we can think of the inverse function. We write this function as

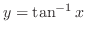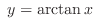
$\displaystyle y = \tan^{-1}{x}$ or $\displaystyle y = \arctan{x}$

and say arctan $x$

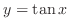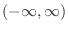The domain of the function $y = tan^{-1}{x}$ is the range of the function $y = \tan{x}$ which is $(-\infty,\infty)$ . The range is 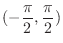$\displaystyle{(-\frac{\pi}{2},\frac{\pi}{2})}$ . Thus we have

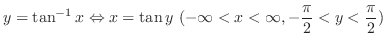
$\displaystyle y = \tan^{-1}{x} \Leftrightarrow x = \tan{y} (-\infty < x < \infty, -\frac{\pi}{2} < y < \frac{\pi}{2})$

The value in the interval $(-\frac{\pi}{2}, \frac{\pi}{2})$ is called principal value of arctan $x$

Example 1..13 Find the value of $x$

satisfies the following.
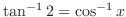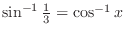
1. $\tan^{-1}{2} = \cos^{-1}{x}$ 2. $\sin^{-1}{\frac{1}{3}} = \cos^{-1}{x}$

SOLUTION 1. Set 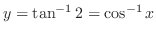$y = \tan^{-1}{2} = \cos^{-1}{x}$ . Then

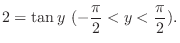
$\displaystyle 2 = \tan{y} (-\frac{\pi}{2} < y < \frac{\pi}{2}).$

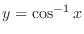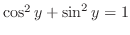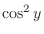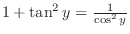Since $y = \cos^{-1}{x}$ , we have $x = \cos{y} \geq 0 (0 \leq y \leq \pi)$ . Now dividing both sides of the identity $\cos^{2}{y} + \sin^{2}{y} = 1$ by $\cos^{2}{y}$ and noting $\tan{y} = \frac{\sin{y}}{\cos{y}}$ , we have $1 + \tan^{2}{y} = \frac{1}{\cos^{2}{y}}$ . Thus,

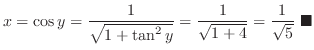
$\displaystyle x = \cos{y} = \frac{1}{\sqrt{1 + \tan^{2}{y}}} = \frac{1}{\sqrt{1 + 4}} = \frac{1}{\sqrt{5}}\ensuremath{ \blacksquare}$

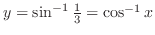2. Let $y = \sin^{-1}{\frac{1}{3}} = \cos^{-1}{x}$ . Then

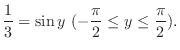
$\displaystyle \frac{1}{3} = \sin{y} (-\frac{\pi}{2} \leq y \leq \frac{\pi}{2}).$

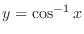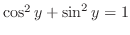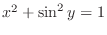Since $y = \cos^{-1}{x}$ , we have $x = \cos{y} \geq 0 (0 \leq y \leq \pi)$ . Substitute this into the identity $\cos^{2}{y} + \sin^{2}{y} = 1$ , we have $x^2 + \sin^{2}{y} = 1$ . Thus,

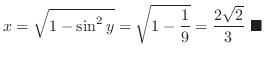
$\displaystyle x = \sqrt{1 - \sin^{2}{y}} = \sqrt{1 - \frac{1}{9}} = \frac{2\sqrt{2}}{3}\ensuremath{ \blacksquare}$

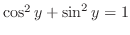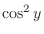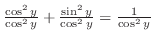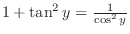
Equivalent of $\tan{x}$ Since $\tan{y} = \frac{\sin{y}}{\cos{y}}$ , by dividing both sides of the identity $\cos^{2}{y} + \sin^{2}{y} = 1$ by $\cos^{2}{y}$ , we obtain $\frac{\cos^{2}{y}}{\cos^{2}{y}} + \frac{\sin^{2}{y}}{\cos^{2}{y}} = \frac{1}{\cos^{2}{y}}$ . Thus, $1 + \tan^{2}{y} = \frac{1}{\cos^{2}{y}}$

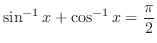Exercise 1..13 Show the identity $\displaystyle{\sin^{-1}{x} + \cos^{-1}{x} = \frac{\pi}{2}}$ holds for all $x$

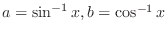SOLUTION Set $a = \sin^{-1}{x}, b = \cos^{-1}{x}$ . Then we have

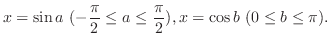
$\displaystyle x = \sin a (-\frac{\pi}{2} \leq a \leq \frac{\pi}{2}), x = \cos b (0 \leq b \leq \pi).$

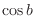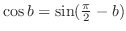Note that we can express $\cos b$ as $\cos b = \sin(\frac{\pi}{2} - b)$ . Then $\sin a = \cos b = \sin(\frac{\pi}{2} - b)$ .

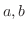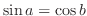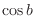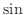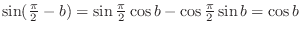To find $a,b$ satisfying $\sin{a} = \cos{b}$ , we express $\cos{b}$ using $\sin$ fuhnction. Note that $\sin(\frac{\pi}{2} - b) = \sin{\frac{\pi}{2}}\cos{b} - \cos{\frac{\pi}{2}}\sin{b} = \cos{b}$ .

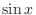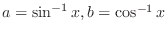Since $-\frac{\pi}{2} \leq \frac{\pi}{2} - b \leq \frac{\pi}{2}$ , the function $\sin{x}$ is one-to-one. Thus $a = \frac{\pi}{2} - b$ . Since $a = \sin^{-1}{x}, b = \cos^{-1}{x}$ , we obtain $\sin^{-1}{x} + \cos^{-1}{x} = \frac{\pi}{2}.$ $\blacksquare$

Exercise A

1.: Evaluate the followings
(a) $\displaystyle{\tan^{-1}{0}}$ (b) $\displaystyle{\sin^{-1}{\frac{1}{2}}}$ (c) $\displaystyle{\sin{\left(\cos^{-1}{\frac{1}{2}}\right)}}$

Exercise B

1.: Find the value of the followings
(a) $\displaystyle{\sin^{-1}{(\frac{-1}{2})}}$ (b) $\displaystyle{\cos^{-1}{(-1)}}$ (c) $\displaystyle{\tan^{-1}{(-1)}}$ (d) $\displaystyle{\tan^{-1}{\sqrt{3}}}$
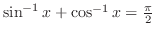2.: Show $\sin^{-1}{x} + \cos^{-1}{x} = \frac{\pi}{2}$ is true for all .
3.: Derive the following formulas
(a) $\displaystyle{\sin^{-1}{(-x)} = - \sin^{-1}{x}}$ (b) $\displaystyle{\cos^{-1}{(-x)} = \pi - \cos^{-1}{x}}$