Next: Random variables Up: Probabilistic model Previous: Definition of probability Contents
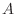 and the event
and the event  are the same. Then we write,
are the same. Then we write, .The event
.The event 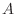 is included in the event
is included in the event  . Then we write
. Then we write
 .
.
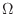
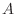 or the event
or the event  can occur and denote by.
can occur and denote by. .
.
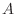 and
and  can occur and denoted by
can occur and denoted by  .
.
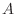 does not occur for
does not occur for 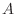 and denoted by
and denoted by
 .
.
 .
.
For the operation of events, the following relational expression holds as in the case of sets.
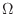 be all events and
be all events and  be empty event. Then
be empty event. Then

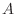 ,
,

 て
て
 |
 |
 |
|
 |
 |
 |
 (DeMorgan's law)
(DeMorgan's law)

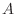 ,
, ,
, ,
,

 that satisfies the following axiom is determined for any event
that satisfies the following axiom is determined for any event 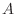 in the sample space
in the sample space 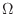 ,
,  is called the probability of the event
is called the probability of the event 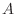 . An event with a possible probability is called a probability event.
. An event with a possible probability is called a probability event.


 , if any two of them are mutually exclusive
, if any two of them are mutually exclusive

Fix event 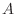 and as a function of event
and as a function of event  , we define
, we define

 when the event
when the event 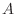 occurs
occurs
If the events
 are mutually exclusive and
are mutually exclusive and

 ,
,


 . Thus we can find
. Thus we can find

1. Let 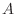 = [Throw the dice 4 times and get a 6 at least once].
= [Throw the dice 4 times and get a 6 at least once].  = [Throw two dice 24 times at the same time and get a 6 at least once].
= [Throw two dice 24 times at the same time and get a 6 at least once].
 .
.
 .
.
2. A patient has complained of certain symptoms. From the experience of doctors, we know that about 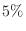 of people in the same age group have cancer when they complain of the condition. On the other hand, a detailed examination shows a positive reaction of
of people in the same age group have cancer when they complain of the condition. On the other hand, a detailed examination shows a positive reaction of  for true cancer patients and a positive reaction of
for true cancer patients and a positive reaction of 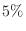 for non-cancer patients. If a patient gives a positive result on the work-up, find the probability that the patient has cancer..
for non-cancer patients. If a patient gives a positive result on the work-up, find the probability that the patient has cancer..
3. Show the following relations..


 and
and  are mutually exclusive,
are mutually exclusive,

1.
(a) The event 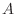 's complementary event
's complementary event
 , in which you throw 4 times and get a 6 at least once, will be thrown 4 times and never get a 6's. Here, the probability of not having a 6 in each time is
, in which you throw 4 times and get a 6 at least once, will be thrown 4 times and never get a 6's. Here, the probability of not having a 6 in each time is
 . Then
. Then


(b) Consider the evemt  in which two dice are thrown 24 times at the same time and both rolls 6 at least once. First, when you throw two dice at the same time, the probability that both will roll a 6 is
in which two dice are thrown 24 times at the same time and both rolls 6 at least once. First, when you throw two dice at the same time, the probability that both will roll a 6 is
 .
.
Now the complement of the event  is
is
 and throw two dice 24 times at the same time, and both of them will not be 6 rolls.
Thus,
and throw two dice 24 times at the same time, and both of them will not be 6 rolls.
Thus,


2 Let  「true cancer patient」,
「true cancer patient」, 「patients came out positive in precision inspection.
Find the probability of being a cancer patient if the patient gives a positive result of the work-up.This can be expressed as follows using conditional probabilities..
「patients came out positive in precision inspection.
Find the probability of being a cancer patient if the patient gives a positive result of the work-up.This can be expressed as follows using conditional probabilities..

 ,
,
 ,
,
 .
Then using,Bayes'theorem,
.
Then using,Bayes'theorem,

 |
 |
 |
|
 |
 |
||
 |
 |
 |
|
 |
 |
3.
 |
 |
 |
|
 |
 |
||
 |
 |
||
 |
 |


 |
 |
 |
|
 |
 |
||
 |
 |
||
 |
 |


(c)
 and
and  are mutually exclusive. Then
are mutually exclusive. Then  and
and  are also mutually exclusive. Thus
are also mutually exclusive. Thus
