Poisson distribution

Those that satisfy the following conditions 1 to 5 are called Poisson process.

Events can occur randomly at any time.
The occurrence of an event in a given time interval is independent of other intervals that do not overlap.
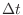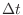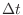The probability of occurrence of an event in a minute time $\Delta t$ decreases in proportion to $\Delta t$ .
As for the probability of occurrence of an event in the minute time $\Delta t$ , the probability that the event occurs more than once in the minute time 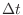$\Delta t$ can be ignored..
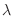The average number of occurrences $\lambda$ of the event during the time 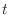is approximately 5 or less.

Let 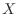$X$ be the number of events that occur in the Poisson process. Then

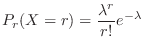
$\displaystyle P_{r}(X = r) = \frac{\lambda^{r}}{r!}e^{-\lambda}$

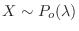and denoted by $X \sim P_{o}(\lambda)$ . Here, 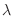$\lambda$ is the average number of events that occur in the Poisson process.

The Poisson process includes scratches on the tape, calls to the switchboard, and broken light bulbs.

Exercise9

1. When the average number of fatalities from traffic accidents is 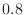$0.8$ per day, what is the next probability?

(a): 0 days of death．
(b): Days of 6 or more deaths．

2.: The average number of particles emitted from a radioactive substance per second is three. Find the probability that 0, 1, 2, 3, 4, 5, or 6 particles will be emitted per second. What is the probability that at least one particle will be released per second?

3.: It is known that one magnetic tape has an average of two scratches per 100 m. At this time, find the probability that there will be no scratches in one roll of tape with a length of 300 m.

Answer

1. If

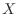is the number of traffic accidents in a day, the event of traffic accidents is a Poisson process. Therefore, the average number of events in a day 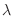$\lambda$ is $0.8$

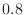The day of 0 deaths means finding $P_{r}(X = 0)$ . Thus

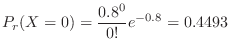
$\displaystyle P_{r}(X = 0) = \frac{0.8^{0}}{0!}e^{-0.8} = 0.4493$

For days with 6 or more deaths, 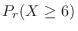$P_{r}(X \geq 6)$ will be calculated. Then

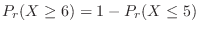
$\displaystyle P_{r}(X \geq 6) = 1 - P_{r}(X \leq 5)$

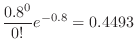$\displaystyle P_{r}(X = 0)$	$\displaystyle =$	$\displaystyle \frac{0.8^{0}}{0!}e^{-0.8} = 0.4493$
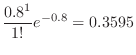$\displaystyle P_{r}(X = 1)$	$\displaystyle =$	$\displaystyle \frac{0.8^{1}}{1!}e^{-0.8} = 0.3595$
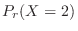$\displaystyle P_{r}(X = 2)$	$\displaystyle =$	$\displaystyle \frac{0.8^{2}}{2!}e^{-0.8} = 0.1438$
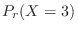$\displaystyle P_{r}(X = 3)$	$\displaystyle =$	$\displaystyle \frac{0.8^{3}}{3!}e^{-0.8} = 0.0383$
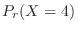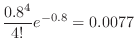$\displaystyle P_{r}(X = 4)$	$\displaystyle =$	$\displaystyle \frac{0.8^{4}}{4!}e^{-0.8} = 0.0077$
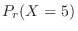$\displaystyle P_{r}(X = 5)$	$\displaystyle =$	$\displaystyle \frac{0.8^{5}}{5!}e^{-0.8} = 0.0012$

Therefore,，

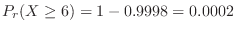
$\displaystyle P_{r}(X \geq 6) = 1 - 0.9998 = 0.0002$

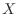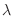2. If $X$ is the number of particles emitted from radioactive material per second, the emission of particles is a Poisson process. Therefore, the average number of occurrences of events in one second $\lambda$ is 3. Than this, The probability that 0 particles will be released per second. Then Similarly，

$\displaystyle P_{r}(X = 1)$	$\displaystyle =$	$\displaystyle \frac{3^{1}}{1!}e^{-3} = 0.1494$
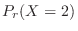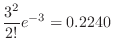$\displaystyle P_{r}(X = 2)$	$\displaystyle =$	$\displaystyle \frac{3^{2}}{2!}e^{-3} = 0.2240$
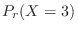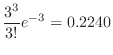$\displaystyle P_{r}(X = 3)$	$\displaystyle =$	$\displaystyle \frac{3^{3}}{3!}e^{-3} = 0.2240$
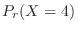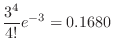$\displaystyle P_{r}(X = 4)$	$\displaystyle =$	$\displaystyle \frac{3^{4}}{4!}e^{-3} = 0.1680$
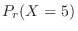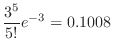$\displaystyle P_{r}(X = 5)$	$\displaystyle =$	$\displaystyle \frac{3^{5}}{5!}e^{-3} = 0.1008$
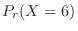$\displaystyle P_{r}(X = 6)$	$\displaystyle =$	$\displaystyle \frac{3^{6}}{6!}e^{-3} = 0.0504$

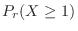Finally, the emission of at least one particle per second is given by $P_{r}(X \geq 1)$ ,

$\displaystyle P_{r}(X \geq 1) = 1 - P_{r}(X < 1) = 1 - P_{r}(X = 0) = 1 - 0.4493 = 0.5407$

3. If 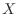$X$ is the number of scratches on the tape, the average number of scratches in 300m is 6, which is $X \sim P_{o}{6}$ . Therefore, the probability that there will be no scratches in one roll of 300m long tape is

$\displaystyle P_{r}(X = 0) = e^{-6}\frac{6^{0}}{0!} = e^{-6} = 0.0025 .$