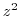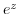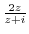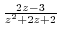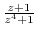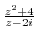Next: Analytic function,holomorphic function Up: Holomorphic function Previous: Holomorphic function Index
 set of points on plane excluding 0
set of points on plane excluding 0
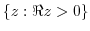
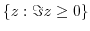

2. Find the limit of the followings.
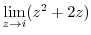
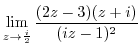
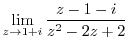

3. Find the point where the following function is not continuous.