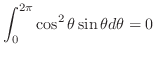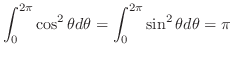Next: 8.6 解答 Up: 演習問題詳解 Previous: 8.4 解答 索引
8.5
1. スカラー場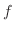 の曲面
の曲面 上での面積分は
上での面積分は
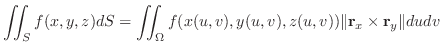
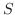 上のベクトル場
上のベクトル場 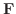 の面積分は次のように2重積分で表わされます.
の面積分は次のように2重積分で表わされます.
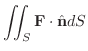 |
 |
 |
|
 |
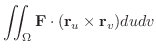 |
||
 |
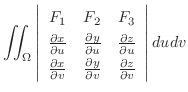 |
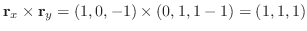
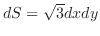 .また,
.また,
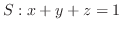 の
の 平面への射影
平面への射影 はV-simpleを用いて表わすと
はV-simpleを用いて表わすと
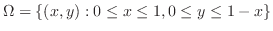
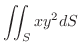 |
 |
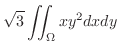 |
|
 |
![$\displaystyle \int_{0}^{1} \int_{0}^{1-x}xy^2 dy dx = \sqrt{3}\int_{0}^{1}\left[\frac{xy^3}{3}\right]_{0}^{1-x} dx$](img5596.png) |
||
 |
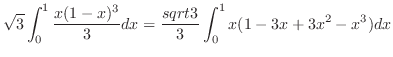 |
||
 |
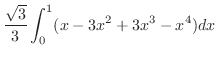 |
||
 |
![$\displaystyle \frac{\sqrt{3}}{3}\left[\frac{x^2}{2} - x^3 + \frac{3x^4}{4} - \frac{x^5}{5}\right]_{0}^{1}$](img5599.png) |
||
 |
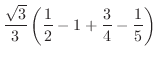 |
||
 |
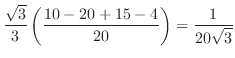 |
(b)
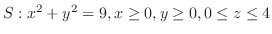 より正射影は
より正射影は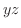 平面または,
平面または,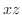 平面となる.そこで,
平面となる.そこで,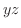 平面に正射影を行うとすると,
平面に正射影を行うとすると,
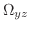 は
は
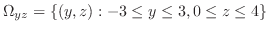
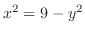 より,
より,
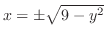 .
.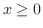 より,
より,
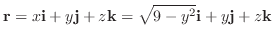
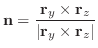
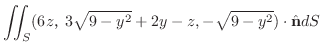 |
 |
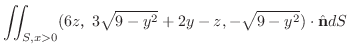 |
|
 |
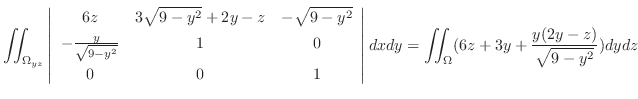 |
||
 |
![$\displaystyle \int_{y=0}^{3}\int_{0}^{4}(6z + 3y + \frac{y(2y - z)}{\sqrt{9 - y...
..._{0}^{3}\left[3z^2 + 3yz + \frac{2y^2z - yz^2/2}{\sqrt{9-y^2}}\right]_{0}^{4}dy$](img5611.png) |
||
 |
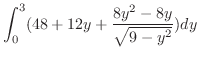 |
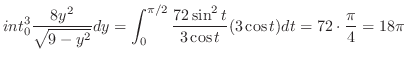
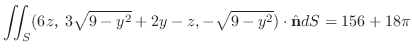

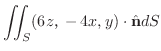 |
 |
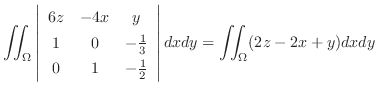 |
|
 |
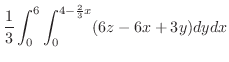 |
||
 |
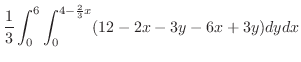 |
||
 |
 |
||
 |
![$\displaystyle \frac{1}{3}\int_{0}^{6}\left[12y - 4x^2\right]_{0}^{4 - \frac{2}{3}x} dx$](img5622.png) |
||
 |
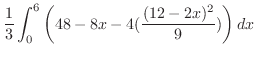 |
||
 |
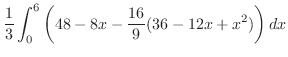 |
||
 |
![$\displaystyle \frac{1}{3}\left[-\frac{16x^3}{27} + \frac{40x^2}{6} - 16x\right]_{0}^{6}$](img5625.png) |
||
 |
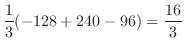 |

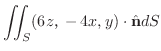 |
 |
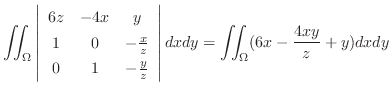 |
|
 |
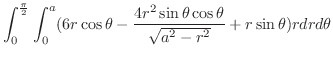 |
||
 |
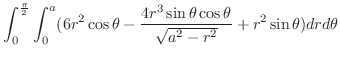 |
||
 |
![$\displaystyle \int_{0}^{\frac{\pi}{2}}\left[2r^3 \cos{\theta} + \frac{r^3}{3}\s...
...}(\frac{2}{3}(a^2 - r^2)^{3/2} - 2a^2 (a^2 - r^2)^{1/2})\right]_{0}^{a} d\theta$](img5633.png) |
||
 |
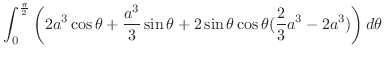 |
||
 |
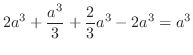 |
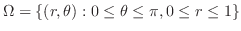
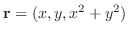 .
.
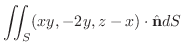 |
 |
 |
|
 |
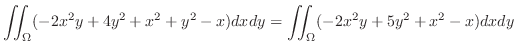 |
||
 |
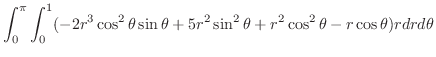 |
||
 |
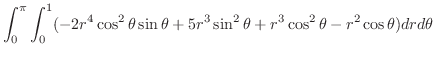 |
||
 |
![$\displaystyle \int_{0}^{\pi}\left[\frac{-2}{5}r^5 \cos^{2}{\theta}\sin{\theta} ...
...rac{r^4}{4} \cos^{2}{\theta} - \frac{r^3}{3}\cos{\theta}\right]_{0}^{1} d\theta$](img5644.png) |
||
 |
![$\displaystyle \int_{0}^{\pi}\left(\frac{-2}{5} \cos^{2}{\theta}\sin{\theta} + \...
...+ \frac{1}{4} \cos^{2}{\theta} - \frac{1}{3}\cos{\theta}\right]_{0}^{1} d\theta$](img5645.png) |