ベクトル関数(vector functions)

実数の部分集合に属する各点に対して，実関数が与えられるとき，1つのベクトルを考えることができます．このベクトル ${\bf F}(t)$ をから $R^{3}$ への1変数ベクトル値関数(vector-valued function)または ベクトル関数(vector function) といい，

$\displaystyle {\bf F}(t) = (x(t),y(t),z(t))$

または

$\displaystyle {\bf F}(t) = x(t)\hat{\bf i} + y(t)\hat{\bf j} + z(t)\hat{\bf k}$

で表わします．

しばしば ${\bf F}(t)$ は幾何学的に実軸から原点と点を結ぶベクトルへの写像として扱われます．

例題 5..1

$\displaystyle{{\bf F}(t) = t\cos{t} \hat{\bf i} + t\sin{t} \hat{\bf j} + t^2 \hat{\bf k}}$ のとき， ${\bf F}(t)$ の軌跡を求めてみましょう．

解 ${\bf F}(t)$ の成分は $x = t\cos{t}, y = t\sin{t}, z = t^2$ であるから，となり， ${\bf F}(t)$ の軌跡は放物面上にあることが分かります． $\blacksquare$

定義 5..1

ベクトル関数 ${\bf F}(t)$ において， $t \rightarrow t_{0}$ のとき， ${\bf F}(t) \rightarrow {\bf L}$ ならば， ${\bf F}(t)$ の極限値は ${\bf L}$ であるといい，次のように表わす．

$\displaystyle \lim_{t \rightarrow t_{0}}{\bf F}(t) = {\bf L}$

極限値の定義は1変数関数のときと同じなので，たぶん連続性の定義も1変数関数のときと同じになると期待するでしょう．実際そのとうりです．

定義 5..2

$\lim_{t \rightarrow t_{0}}{\bf F}(t) = {\bf F}(t_{0})$ が成り立つとき，ベクトル関数 ${\bf F}(t)$ は $t = t_{0}$ で連続であるという．また，区間

のすべての

で連続なとき， ${\bf F}(t)$ は区間

で連続であるといい， ${\bf F}(t) \in C[a,b]$ と表わす．

このように1変数関数における様々な定義はベクトル関数へと継承されます．

定義 5..3

ベクトル関数 ${\bf F}(t)$ は $t = t_{0}$ において，

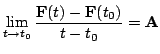
$\displaystyle \lim_{t \rightarrow t_{0}}\frac{{\bf F}(t) - {\bf F}(t_{0})}{t - t_{0}} = {\bf A}$

が存在するとき $t = t_{0}$ で微分可能(differentiable) であるという．また，この極限値 ${\bf A}$ を点 $t_{0}$ における微分係数といい， ${\bf F}^{\prime}(t_{0})$ で表わす．

次の節で学びますがベクトル ${\bf F}^{\prime}(t_{0})$ の方向は， ${\bf F}(t)$ によって描かれる曲線の $t = t_{0}$ での接線方向になります．図5.1参照

ベクトルの和やスカラー倍がそれぞれの対応する成分の和やスカラー倍で定義されたように，ベクトル関数の極限値，微分係数，不定積分の計算は，ベクトル関数の成分の極限値，微分係数，不定積分より求めることができます．

定理 5..1

${\bf F}(t) = (x(t),y(t),z(t))$ とすると，次のことが成り立つ．

$\displaystyle{(a) \lim_{t \rightarrow t_{0}}{\bf F}(t) = (\lim_{t \rightarrow t_{0}}x(t),\lim_{t \rightarrow t_{0}}y(t),\lim_{t \rightarrow t_{0}}z(t))}$

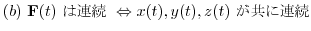
$\displaystyle{(b) \ {\bf F}(t) \ \mbox{は連続} \ \Leftrightarrow x(t), y(t), z(t) \ \mbox{が共に連続}}$

$\displaystyle{(c) {\bf F} \in C'[a,b] \Rightarrow {\bf F}^{\prime}(t_{0}) = (x^{\prime}(t_{0}),y^{\prime}(t_{0}),z^{\prime}(t_{0}))}$

$\displaystyle{(d) {\bf F} \in C[a,b] \Rightarrow \int_{a}^{b}{\bf F}(t) dt = (\int_{a}^{b} x(t)dt, \int_{a}^{b} y(t)dt, \int_{a}^{b} z(t)dt)}$

証明 (a)

$\displaystyle \vert{\bf F}(t) - {\bf L}\vert$	$\displaystyle =$	$\displaystyle \vert(x(t),y(t),z(t)) - (l_{1},l_{2},l_{3})\vert$
	$\displaystyle =$	$\displaystyle \vert(x(t) - l_{1}, y(t) - l_{2}, z(t) - l_{3})\vert$
	$\displaystyle =$	$\displaystyle \sqrt{(x(t) - l_{1})^2 + (y(t) - l_{2})^2 + (z(t) - l_{3})^2 }$

より， $x(t) \rightarrow l_{1}, y(t) \rightarrow l_{2}, z(t) \rightarrow l_{3}$ ならば， ${\bf F}(t) \rightarrow {\bf L}$ ．また， ${\bf F}(t) \rightarrow {\bf L}$ ならば，

$\displaystyle \sqrt{(x(t) - l_{1})^2 + (y(t) - l_{2})^2 + (z(t) - l_{3})^2 } \rightarrow 0$

ここで，平方根の中はすべて2乗の和であることに注意すると

$\displaystyle (x(t) - l_{1})^2 \rightarrow 0, (y(t) - l_{2})^2 \rightarrow 0, (z(t) - l_{3})^2 \rightarrow 0$

となる．よって $x(t) \rightarrow l_{1}, y(t) \rightarrow l_{2}, z(t) \rightarrow l_{3}$ より，

$\displaystyle \lim_{t \rightarrow t_{0}}{\bf F}(t) = (\lim_{t \rightarrow t_{0}... ...ghtarrow t_{0}}y(t),\lim_{t \rightarrow t_{0}}z(t)) \ensuremath{ \blacksquare}$

(b)，(c)，(d)の証明は演習問題にまわします．

例題 5..2

$\displaystyle{{\bf F}(t) = (t^{2},t,t^{3})}$ のとき， ${\bf F}^{\prime}(t)$ を求めてみましょう．

解それぞれの成分を微分することにより

$\displaystyle {\bf F}^{\prime}(t) = (2t,1,3t^{2}) \ensuremath{ \blacksquare}$

${\bf F}(t),{\bf G}(t)$ がについて微分可能なベクトル関数ならば，次のことが成り立ちます．

$\displaystyle (1)$		$\displaystyle ({\bf F}(t) \pm {\bf G}(t))^{\prime} = {\bf F}^{\prime}(t) \pm {\bf G}^{\prime}(t)$
$\displaystyle (2)$		$\displaystyle (c {\bf F}(t))^{\prime} = c {\bf F}^{\prime}(t)$
$\displaystyle (3)$		$\displaystyle ({\bf F}(t) \cdot {\bf G}(t))^{\prime} = {\bf F}^{\prime}(t) \cdot {\bf G}(t) + {\bf F}(t) \cdot {\bf G}^{\prime}(t)$
$\displaystyle (4)$		$\displaystyle ({\bf F}(t) \times {\bf G}(t))^{\prime} = {\bf F}^{\prime}(t) \times {\bf G}(t) + {\bf F}(t) \times {\bf G}^{\prime}(t)$

例題 5..3

ベクトル関数 ${\bf F}(t)$ の大きさ $\Vert{\bf F}(t)\Vert$ が定数のとき， ${\bf F}(t)$ と ${\bf F}^{\prime}(t)$ は全ての

において直交することを示してみましょう．

解 $\Vert{\bf F}(t)\Vert = c$ より， $\Vert{\bf F}(t)\Vert^2 = {\bf F}(t) \cdot {\bf F}(t) = c^{2}$ . よってベクトル関数の微分法より

$\displaystyle ({\bf F}(t) \cdot {\bf F}(t))^{\prime} = 2{\bf F}^{\prime}(t) \cdot {\bf F}(t) = 0$

したがって，内積が0より ${\bf F}(t)$ と ${\bf F}^{\prime}(t)$ は直交します． $\blacksquare$

確認問題

1.

次の問に答えよう．

(a) $\displaystyle{{\bf F}(t) = (\cos{t}, \sin{t})}$ のとき， $\displaystyle{{\bf F}^{\prime}(t)}$ と $\displaystyle{\Vert{\bf F}^{\prime}(t)\Vert}$ を求めよう．

(b) $\displaystyle{{\bf F}(t) = (1+2t, 3-t,2+3t)}$ のとき， $\displaystyle{{\bf F}^{\prime}(t)}$ と $\displaystyle{\Vert{\bf F}^{\prime}(t)\Vert}$ を求めよう．

(d) $\displaystyle{{\bf F}'(t) = (e^{t}, \sqrt{2}, e^{-t})}$ のとき， ${\bf F}(t)$ を求めよう．

演習問題

1.

次の問に答えよう．

(a) $\displaystyle{{\bf F}(t) = (\sin{t}, \cos^{2}{t}, t^{2})}$ のとき， $\displaystyle{{\bf F}^{\prime}(t)}$ を求めよう．

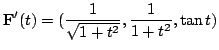(b) $\displaystyle{{\bf F}^{\prime}(t) = (\frac{1}{\sqrt{1 + t^2}}, \frac{1}{1 + t^2}, \tan{t})}$ のとき， ${\bf F}(t)$ を求めよう．

$\displaystyle ({\bf F} \cdot {\bf G})^{\prime} = {\bf F}^{\prime} \cdot {\bf G} + {\bf F} \cdot {\bf G}^{\prime}$

が成り立つことを証明しよう．

(d) ${\bf F}(t),{\bf G}(t)$ がについて微分可能なベクトル関数のとき，

$\displaystyle ({\bf F} \times {\bf G})^{\prime} = {\bf F}^{\prime} \times {\bf G} + {\bf F} \times {\bf G}^{\prime}$

が成り立つことを示そう．

(e) 定理5.1(b)，(c)，(d)を証明しよう．