Gradient and directional derivative

When the real number $\phi({\rm P})$ corresponds to each point ${\rm P}$ in the space area 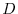The three-variable function $\phi({\rm P})$ is called scalar field on 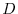. Similarly, in the space area 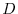, when the vector $\boldsymbol{F}({\rm P})$ corresponds to each point ${\rm P}$ , the three-variable vector function $\boldsymbol{F}({\rm P}))$ is called vector field on 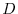. 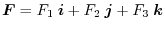Let the component of the vector $\boldsymbol{F}$ be $\boldsymbol{F} = F_{1}\:\boldsymbol{i} + F_{2}\:\boldsymbol{j} + F_{3}\:\boldsymbol{k}$ . Then

$\displaystyle \boldsymbol{F}({\rm P}) = F_{1}(x,y,z)\:\boldsymbol{i} + F_{2}(x,y,z)\:\boldsymbol{j} + F_{3}(x,y,z)\:\boldsymbol{k}$

Also, if $F_{1}, F_{2}, F_{3}$ are continuous，then $\boldsymbol{F}$ is said to be continuous.

Example 3..1

Graph the vector field $\displaystyle{\boldsymbol{F}(x,y) = -y\:\boldsymbol{i} + x\:\boldsymbol{j}}$ ．

Answer Since the vector corresponds to the point 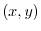on the 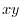plane, a four-dimensional space is required to draw a graph. Unfortunately, a four-dimensional space cannot be prepared, so the vector field is expressed using the following method. First, select the point $(x_{0}, y_{0})$ on the 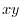plane, and then set the vector $\boldsymbol{F}(x_{0}, y_{0})$ at that point. Draw the point $(x_{0}, y_{0})$ as the starting point.

$\displaystyle \boldsymbol{F}(1,0) = \boldsymbol{j},\ \boldsymbol{F}(2,-1) = \bo... ... + 2\boldsymbol{j},\ \boldsymbol{F}(3,2) = -2\:\boldsymbol{i} + 3\boldsymbol{j}$

Then we have the following graph 3.1.

**Figure 3.1:** vector field
$\begin{figure}\begin{center} \includegraphics[width=5cm]{VECANALFIG/Fig8-2-1.eps} \end{center}\vskip -1cm \end{figure}$

If you look at the figure 3.1, you will notice that the vector is a tangent to a curve.．This curve is called streamlines or lines of force. Generally, when $\boldsymbol{F}$ represents the velocity of a fluid, the curve drawn along the flow is called a streamline, and when $\boldsymbol{F}$ represents a magnetic field, it is along the direction of the magnetic field. The drawn curve is called the magnetic field line.．Similarly, when $\boldsymbol{F}$ represents an electric field, the curve drawn along the direction of the electric field is drawn along the power line, and when $\boldsymbol{F}$ represents the electromagnetic field, it is drawn along the direction of the electromagnetic field. The curved line is called an electromagnetic force line.

Bring a magnet to the sandbox and collect iron sand. If you sprinkle this iron sand on paper and place a U magnet under the paper, the iron sand will line up along the lines of magnetic force, and you may have observed that the stronger the magnetic field, the more iron sand is attached. Let us consider these phenomena here.．

Electric field

If the distance from the charge to the point P is and the unit vector from to P is $\boldsymbol{r}_{0}$ , the electric field at the point P is given by the following equation.

$\displaystyle {\bf E} = \frac{1}{4\pi \epsilon_{0}}\frac{q}{r^2} \boldsymbol{r}_{0}$

Note that， $\epsilon_{0} = 8.85418782\times 10^{-12} [{\rm s^2C^2/kgm^2}]$ is called the permittivity of vacuum

Universal gravitational field

$\boldsymbol{F}$ for a universal gravitational field (generally called universal gravitational force) in which an object with a substance amount of at the origin acts on an object with a substance amount of at a point P 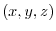Then

$\displaystyle \boldsymbol{F} = - \frac{\kappa Mm}{r^3}(x,y,z) = - \frac{\kappa Mm}{\vert\boldsymbol{r}\vert^3}\boldsymbol{r}$

Gradient

Here, for a scalar field defined in an area of space，consider vector field ${\rm grad} f$ defined by

$\displaystyle \nabla f = f_{x}\:\boldsymbol{i} + f_{y}\:\boldsymbol{j} + f_{z}\:\boldsymbol{k}$

Here, $\nabla f$ can be seen as an operator applied to by

$\displaystyle \nabla = \frac{\partial}{\partial x}\:\boldsymbol{i} + \frac{\partial}{\partial y}\:\boldsymbol{j} + \frac{\partial}{\partial z}\:\boldsymbol{k}$

This is called gradient of .

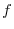For the scalar field 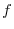, the curved surface defined by (c constant) is the level surface of the scalar field 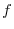. and the group of coordinating surfaces obtained by changing the value of is called the coordinating surface group.

Example 3..2

Show that the gradient through the point $\displaystyle{(x_{0},y_{0},z_{0})}$ is orthogonal to the level surface through the point $\displaystyle{(x_{0},y_{0},z_{0})}$ .

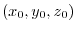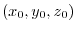Answer Let the level surface through the point $(x_{0},y_{0},z_{0})$ be

$\displaystyle f(x,y,z) = c$

Let any curve that passes through the point $(x_{0},y_{0},z_{0})$ on this level surface be

$\displaystyle \boldsymbol{r} = \boldsymbol{r}(t) = x(t)\:\boldsymbol{i} + y(t)\:\boldsymbol{j} + z(t)\:\boldsymbol{k}$

Then $f(\boldsymbol{r}(t)) = f(x(t),y(t),z(t)) = c$ . Thus differentiate both sides by .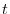

$\displaystyle f_{x}x_{t} + f_{y}y_{t} + f_{z}z_{t} = (f_{x},f_{y},f_{z})\cdot(x_{t},y_{t},z_{t}) = \nabla f \cdot\frac{d \boldsymbol{r}}{dt} = 0$

Therefore, the gradient is orthogonal to the level surface because it is orthogonal to the tangents of the curves on all level surfaces.

Directional derivatives

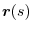Let the unit vector ${\ bf u}$ be the directional unit vector at point P. Also, a straight line passing through the point P and having ${\ bf u}$ as the direction vector is represented by $\boldsymbol{r}(s)$ using the distance 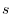from the point P. Then, at the point P, the directional derivative of the scalar field 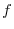in the ${\ bf u}$ direction is given by

$\displaystyle \frac{{\partial f(\boldsymbol{r}(s))}}{\partial u} = \lim_{s\to 0}\frac{f(p+s{\bf u})-f(p)}{s}$

Therefore,

$\displaystyle \frac{\partial f(\boldsymbol{r}(s))}{\partial u}$	$\displaystyle =$	$\displaystyle \lim_{s\to 0}\frac{f(p+s{\bf u})-f(p)}{s} = \frac{d}{ds}f(x(s),y(s),z(s)) \mid_{s=0}$
	$\displaystyle =$	$\displaystyle f_{x}x_{s} + f_{y}y_{s} + f_{z}z_{s} = \nabla f \cdot\frac{d \boldsymbol{r}}{ds} = \nabla f \cdot{\bf u}$

Example 3..3

Find the unit normal vector orthogonal to the surface $\displaystyle{z^{2} = x^{2} + 2y^{2}}$ at and find the directional derivative in the direction of and the equation of tangent plane.

Answer Let

$\displaystyle f(x,y,z)=x^{2}+2y^{2}-z^{2}$

Then the level surface is . Also, ， the normal vector is given by $\nabla f$ . Thus

$\displaystyle \nabla f = (2x, 4y, -2z), \nabla f \mid_{(1,2,3)} = (2,8,-6)$

Therefore,

$\displaystyle \boldsymbol{n} = \frac{\nabla f}{\vert\nabla f\vert} = \frac{(2,8,-6)}{\sqrt{4+64 +36}} = \frac{(2,8,-6)}{\sqrt{104}}$

Next, find the directional unit vector to find the directional derivative in the direction at the point . $\displaystyle{{\bf u} = \frac{(1,3,-1)}{\sqrt{11}}}$ ．Then the directional derivative is

$\displaystyle \frac{\partial f}{\partial u} = \nabla f \cdot{\bf u} = (2,8,-6) \cdot\frac{(1,3,-1)}{\sqrt{11}} = \frac{32}{\sqrt{11}}$

Also, the equation of the tangent plane is

$\displaystyle 2(x-1) + 8(y-2) - 6(z - 3) = 0$ that is $\displaystyle 2x + 8y - 6z = 0$

Example 3..4

Find the streamline of the vector field $\displaystyle{\boldsymbol{F}(x,y) = -y\:\boldsymbol{i} + x\:\boldsymbol{j}}$ ．

Answer Let be a streamline equation. Then $\nabla f(x,y) = f_{x}\:\boldsymbol{i} + f_{y}\:\boldsymbol{j}$ expresses the normal vector of .

$\displaystyle \nabla f(x,y) \cdot\boldsymbol{F}(x,y) = 0 \ Thus, \ (f_{x}\:\bol... ...{i} + f_{y}\:\boldsymbol{j}) \cdot(-y\:\boldsymbol{i} + x\:\boldsymbol{j}) = 0$

This implies that， $-y f_{x} + xf_{y} = 0$ ．Note that the slope of the tangent line of is

$\displaystyle f_{x}dx + f_{y} dy = 0$ implies $\displaystyle \frac{dy}{dx} = - \frac{f_{x}}{f_{y}}$

Then

$\displaystyle \frac{dy}{dx} = - \frac{f_{x}}{f_{y}} = - \frac{x}{y}$

implies ．Thus $f_{x} = x, f_{y} = y$ and

$\displaystyle f(x,y) = \int f_{x}dx = \frac{x^2}{2} + c(y) \$

(3.1)

Next differentiate the equation 3.1 with respect to . Then

$\displaystyle f_{y} = c'(y) = y$ implies $\displaystyle c(y) = \frac{y^2}{2}$

Therefore, the equation of the streamline is

$\displaystyle f(x,y) = \frac{x^2}{2} + \frac{y^2}{2} = c$

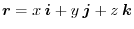Potential Let the position vector of the point P be $\boldsymbol{r} = x\boldsymbol{i} + y\boldsymbol{j} + z\boldsymbol{k}, r = \vert\boldsymbol{r}\vert = \sqrt{x^2 + y^2 + z^2}$ Then there are many things that are inversely proportional to the distance, such as the magnitude of universal gravitation and the intensity of light. These can be set by

$\displaystyle f(x,y,z) = \frac{c}{r} = \frac{c}{\sqrt{x^2 + y^2 + z^2}}$

Here we find the gradient of .

$\displaystyle f_{x}$	$\displaystyle =$	$\displaystyle c\frac{\partial}{\partial x}(x^{2}+y^{2}+x^{2})^{-\frac{1}{2}} = ... ...^{2}+y^{2}+z^{2})^{-\frac{3}{2}}(2x) = \frac{-cx}{\vert\boldsymbol{r}\vert^{3}}$
$\displaystyle f_{y}$	$\displaystyle =$	$\displaystyle \frac{-cy}{\vert\boldsymbol{r}\vert^{3}}$
$\displaystyle f_{z}$	$\displaystyle =$	$\displaystyle \frac{-cz}{\vert\boldsymbol{r}\vert^{3}}$

implies

$\displaystyle \nabla f = (f_{x},f_{y},f_{z}) = (\frac{-cx}{r^{3}},\frac{-cy}{r^{3}},\frac{-cz}{r^{3}}) = -c \frac{\boldsymbol{r}}{r^3}$

Therefore， $\nabla f$ is equal to the vector field.

Definition 3..1

If vector field $\boldsymbol{F}$ is the gradient of , in other words, $\boldsymbol{F} = -\nabla f$ . Then $\boldsymbol{F}$ is called conservative field．Also，the scalar field is called scalar potential of $\boldsymbol{F}$ and $\boldsymbol{F}$ is said to have a scalar potential of ．

Example 3..5

Let the position vector of ${\rm P}(x,y,z)$ be $\boldsymbol{r}= x\:\boldsymbol{i} + y\:\boldsymbol{j} + z\:\boldsymbol{k}$ ，and vector field be $\displaystyle{\boldsymbol{F} = -\frac{\boldsymbol{r}}{\vert\boldsymbol{r}\vert}}$ . Then show that this vector field is a conservative field in any area except at origin and

$\displaystyle f(x,y,z) = \vert\boldsymbol{r}\vert = (x^{2}+y^{2}+z^{2})^{1/2}$

is the scalar potential of $\boldsymbol{F}$ .

Answer

$\displaystyle f_{x}$	$\displaystyle =$	$\displaystyle \frac{\partial}{\partial x}(x^{2}+y^{2}+x^{2})^{\frac{1}{2}} = \f... ...}{2}(x^{2}+y^{2}+z^{2})^{-\frac{1}{2}}(2x) = \frac{x}{\vert\boldsymbol{r}\vert}$
$\displaystyle f_{y}$	$\displaystyle =$	$\displaystyle \frac{y}{\vert\boldsymbol{r}\vert}$
$\displaystyle f_{z}$	$\displaystyle =$	$\displaystyle \frac{z}{\vert\boldsymbol{r}\vert}$

implies $-\nabla f=(f_{x},f_{y},f_{z}) = \boldsymbol{F}$ ． Thus， $\boldsymbol{F}$ is a conservative field.

$\displaystyle f(x,y,z) = {\vert\boldsymbol{r}\vert} = (x^{2}+y^{2}+z^{2})^{1/2}$

is the scalar potential of $\boldsymbol{F}$ .

Example 3..6

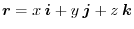For the vector field $\boldsymbol{r}= x\:\boldsymbol{i} + y\:\boldsymbol{j} + z\:\boldsymbol{k}$ and the scalar field $r = \vert\boldsymbol{r}\vert = \sqrt{x^2 + y^2 + z^2}$ , show the followings:．

$\displaystyle (1)\ \nabla r = \frac{\boldsymbol{r}}{r}\ \hskip 1cm (2)\ \nabla r^{n} = nr^{n-2}\boldsymbol{r}$

Answer (1)

$\displaystyle \nabla r = (\frac{\partial}{\partial x}\:\boldsymbol{i} + \frac{\... ...ol{j} + 2z\:\boldsymbol{k}}{2\sqrt{x^2 + y^2 + z^2}} = \frac{\boldsymbol{r}}{r}$

(2)

$\displaystyle \nabla r^{n}$	$\displaystyle =$	$\displaystyle \frac{\partial}{\partial x}(r^{n})\: \boldsymbol{i} + \frac{\part... ...y}(r^{n})\: \boldsymbol{j} + \frac{\partial}{\partial z}(r^{n})\:\boldsymbol{k}$
	$\displaystyle =$	$\displaystyle \frac{\partial}{\partial r}(r^{n})\frac{\partial r}{\partial x}\:... ...frac{\partial }{\partial r}(r^{n})\frac{\partial r}{\partial x}\:\boldsymbol{k}$
	$\displaystyle =$	$\displaystyle nr^{n-1} \left(\frac{\partial r}{\partial x}\boldsymbol{i} + \fra... ...= nr^{n-1}\nabla r = nr^{n-1} \frac{\boldsymbol{r}}{r} = nr^{n-2}\boldsymbol{r}$

Exercise3.2

1.

For the scalar field $\phi = x^2 z + e^{y/x},\ \psi = 2z^2 y - xy^2$ , find the followings: (1) $\nabla \phi, \ \nabla \psi$

(2) the value $\nabla (\phi\psi)_{P}$ of $\nabla(\phi \psi)$ at P.

2.

Find the directional derivative in the direction of ${\bf u} = \frac{1}{7}(2\boldsymbol{i} - 3\boldsymbol{j} + 6\boldsymbol{k})$ of $\phi = 4xz^3 - 3x^2yz$ at P.

3.

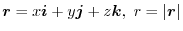Let $\boldsymbol{r} = x\boldsymbol{i} + y\boldsymbol{j} + z\boldsymbol{k},\ r = \vert\boldsymbol{r}\vert$ . Then find ${\bf A, B}$ .．

(1) $\boldsymbol{A} = \nabla\left(2r^2 - 4\sqrt{r} + \frac{6}{3\sqrt{r}}\right)$ ,(2) $\boldsymbol{B} = \nabla(r^2 e^{-r})$

4.

Find the unit normal vector ${\bf n}$ of at P．

5.

For any scalar fields $\phi, \psi$ , show the followings.

$\displaystyle \nabla \left(\frac{\phi}{\psi}\right) = \frac{\psi \nabla \phi - \phi \nabla \psi}{\phi^2}$

6.

Let the distance of P, Q $(\xi, \eta, \zeta)$ be . Then prove the following for the differential operator

$\displaystyle \nabla_{P} = \boldsymbol{i}\frac{\partial }{\partial x} + \boldsy... ...}\frac{\partial}{\partial \eta} + \boldsymbol{k}\frac{\partial}{\partial \zeta}$

$\displaystyle{(1)\ \nabla_{Q}r = - \nabla_{P} r\hskip 3cm (2)\ \nabla_{Q}(\frac{1}{r}) = - \nabla_{P}(\frac{1}{r})}$