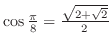Next: Graph of Trig Functions Up: Functions Previous: Functions of single variable Contents Index
 on the unit circle
on the unit circle
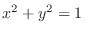 . Now set
. Now set
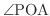 as
as 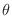 . The angle
. The angle
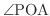 for which the arclength of AP is 1 is called 1 radian and denoted by 1radradian..
for which the arclength of AP is 1 is called 1 radian and denoted by 1radradian..
Then angle 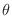 is positive if you measure counter clockwise from the positive
is positive if you measure counter clockwise from the positive  -axis.
-axis.
NOTE
Suppose that
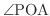 is
is 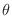 degree and
degree and  radian. Then
radian. Then
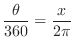
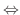 Degree
Degree
 Also, basic angles are measured in degrees and radians to give
Also, basic angles are measured in degrees and radians to give
| Degree | 0 | 30 | 45 | 60 | 90 | 120 | 150 | 180 | 360 |
| Radian | 0 |
 |
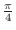 |
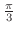 |
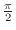 |
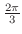 |
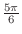 |
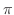 |
 |
Trigonometric Functions
Suppose that
 . Then the following functions of
. Then the following functions of 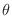 are called Trigonometric functions.
are called Trigonometric functions.

NOTE As 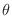 chages the value, the point P
chages the value, the point P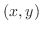 and the shape of the right triangle
and the shape of the right triangle 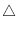 OPH changes.
OPH changes.
 2.
2.
 3.
3.
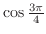 4.
4.
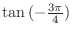
SOLUTION 1. Draw a unit circle with the origin O and draw a line OP with
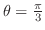 . Then the value of
. Then the value of  coordinate of P is equal to
coordinate of P is equal to
 .
.
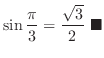
 coordinate of P where
coordinate of P where
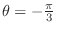 is equal to
is equal to
 . Thus we have
. Thus we have
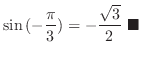
3. The value of  coordinate of P where
coordinate of P where
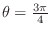 is equal to
is equal to
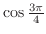 . Thus we have
. Thus we have
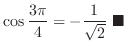
4. Stretch the line OP with
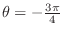 so that
so that  coordinate is -1. Then the ratio of the values of
coordinate is -1. Then the ratio of the values of  coordinate and
coordinate and  coordinate is
coordinate is
 . Thus we have
. Thus we have
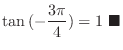
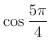 2.
2.
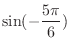 3.
3.
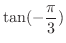
SOLUTION 1. Draw a unit circle with the origin O and draw a line OP with
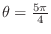 . Then the value of
. Then the value of  coordinate of P is equal to
coordinate of P is equal to
 . Thus we have
. Thus we have
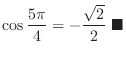
 . Then the value of
. Then the value of  coordinate of P is equal to
coordinate of P is equal to
 . Thus we have
. Thus we have

3. Stretch the line OP with
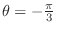 so that
so that  coordinate is 1. Then the ratio of the values of
coordinate is 1. Then the ratio of the values of  coordinate and
coordinate and  coordinate is
coordinate is
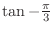 . Thus we have
. Thus we have

Basic Trig Identities
For all
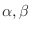 ,
,
1.
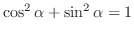
2.
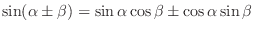
3.
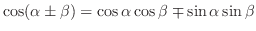
NOTE 1. Consider the point P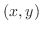 on the unit circle. Then
on the unit circle. Then

2. ,3. Look at the figure, you will see
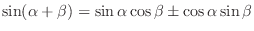
2. ,3. Write
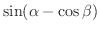 as
as
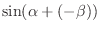 and note that
and note that
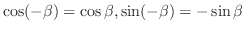 .
.
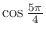 .
.
SOLUTION
 |
 |
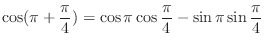 |
|
 |
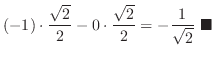 |
Value of Trig Fct Using the know trig values to create the new one.
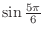 .
.SOLUTION
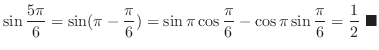
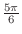
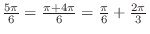
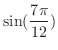 .
.
SOLUTION
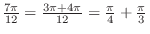 . Now using trigonometric addition formula, we have
. Now using trigonometric addition formula, we have
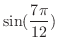 |
 |
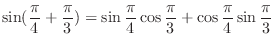 |
|
 |
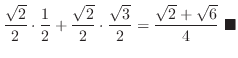 |
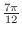
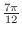 is equivalent to
is equivalent to 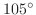 and
and
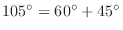
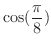 .
.
SOLUTION
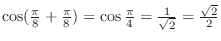 . Using trigonometric addition formula
. Using trigonometric addition formula
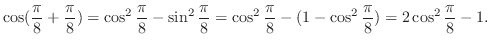
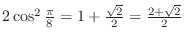 .
.
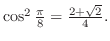 Here,
Here,
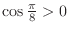 , we have
, we have