1.7 Answer

(a)For any number 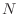$N$

is chosen, there is a number $n$

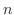so that 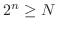$2^{n} \geq N$ . Thus, it is unbounded.

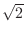(b) $a_{n}$ represents $\sqrt{2}$ to the $n$

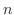th decimal place. So even as $n$

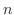gets larger, it can not be greater than 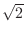$\sqrt{2}$ . Thus it is bounded.

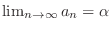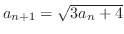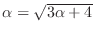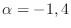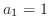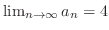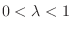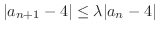(a)Let $\lim_{n \to \infty}a_{n} = \alpha$ . Then $a_{n+1} = \sqrt{3a_{n} + 4}$ . Thus $\alpha = \sqrt{3\alpha + 4}$ ． Now square both sides, we have $\alpha^2 = 3 \alpha + 4$ . Thus, $\alpha = -1,4$ ． By the initial condition $a_{1} = 1$ , then $\alpha = 4$ ．We next show that $\lim_{n \to \infty}a_{n} = 4$ ． By the theorem1．13, we need to show that the exists $0 < \lambda < 1$ so that $\vert a_{n+1} - 4\vert \leq \lambda \vert a_{n} - 4\vert$ .

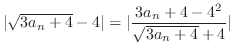$\displaystyle \vert a_{n+1} - 4\vert$	$\displaystyle =$	$\displaystyle \vert\sqrt{3a_{n} + 4} - 4\vert = \vert\frac{3a_{n} + 4 - 4^2}{\sqrt{3a_{n} + 4} + 4}\vert$
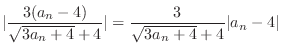	$\displaystyle =$	$\displaystyle \vert\frac{3(a_{n} - 4)}{\sqrt{3a_{n} + 4} + 4}\vert = \frac{3}{\sqrt{3a_{n} + 4} + 4} \vert a_{n} - 4\vert$

(b) $\displaystyle{a_{3} = 2^{\frac{1}{2}}, a_{4} = 2^{\frac{3}{4}}, a_{5} = 2^{\frac{5}{8}}, \ldots , a_{n} = 2^{\frac{2(n-2) - 1}{2^{n-2}}}}$ ． Thus, $\lim_{n \to \infty} a_{n} = 1$

(a) $\displaystyle{\lim_{n \to \infty}(1 - \frac{1}{n^2})^n = \lim_{n \to \infty}(1 + \frac{1}{n})^{n}(1 - \frac{1}{n})^{n} = e \frac{1}{e} = 1}$

$\displaystyle \lim_{n \to \infty}(1 + \frac{2}{n})^{n} = \lim_{m \to \infty}[(1 + \frac{1}{m})^{m}]^{2} = e^{2}$

(c)Using $\displaystyle{\lim_{n \to \infty}\left\vert\frac{a_{n+1}}{a_{n}}\right\vert < 1 \leftrightarrow \lim_{n \to \infty} a_{n} = 0 }$ , we have

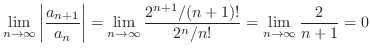
$\displaystyle \lim_{n \to \infty} \left\vert\frac{a_{n+1}}{a_{n}}\right\vert = ... ... \infty}\frac{2^{n+1}/(n+1)!}{2^{n}/n!} = \lim_{n \to \infty}\frac{2}{n+1} = 0$

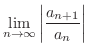(d) Using $\displaystyle{\lim_{n \to \infty}\left\vert\frac{a_{n+1}}{a_{n}}\right\vert < 1 \leftrightarrow \lim_{n \to \infty} a_{n} = 0 }$ , we have

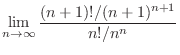$\displaystyle \lim_{n \to \infty} \left\vert\frac{a_{n+1}}{a_{n}}\right\vert$	$\displaystyle =$	$\displaystyle \lim_{n \to \infty}\frac{(n+1)!/(n+1)^{n+1}}{n!/n^{n}}$
	$\displaystyle =$	$\displaystyle \lim_{n \to \infty}\frac{n+1}{n+1}\left(\frac{n}{n+1}\right)^{n}$
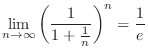	$\displaystyle =$	$\displaystyle \lim_{n \to \infty}\left(\frac{1}{1+\frac{1}{n}}\right)^{n} = \frac{1}{e}$